不同地震强度下新型装配式锚索框架梁加固边坡抗震性能分析

戴轩; 马云祥; 魏少伟; 魏培勇; 霍海峰; 蔡德钩; 李朝

doi:10.11779/CJGE2023S20019

不同地震强度下新型装配式锚索框架梁加固边坡抗震性能分析 English Version

戴轩^1,,
马云祥¹,
魏少伟^{2, 3, ,},
魏培勇^{2, 3},
霍海峰¹,
蔡德钩^{2, 3},
李朝¹

1.
中国民航大学交通科学与工程学院, 天津 300300
2.
中国铁道科学研究院集团有限公司铁道建筑研究所, 北京 100081
3.
北京铁科特种工程技术有限公司, 北京 100081

基金项目:

中国民航大学科研启动基金项目 2020KYQD39

详细信息

作者简介:
戴轩(1988—)，男，博士，副教授，主要从事岩土工程和机场工程等方面的教学和科研工作。E-mail: tianjindaixuan@126.com

通讯作者:
魏少伟, E-mail: 752929275@qq.com

中图分类号: TU432
计量
- 文章访问数: 100
- HTML全文浏览量: 17
- PDF下载量: 22
出版历程
- 收稿日期: 2023-11-29
- 网络出版日期: 2024-04-19
- 刊出日期: 2023-11-30

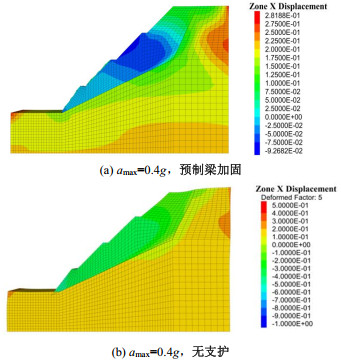
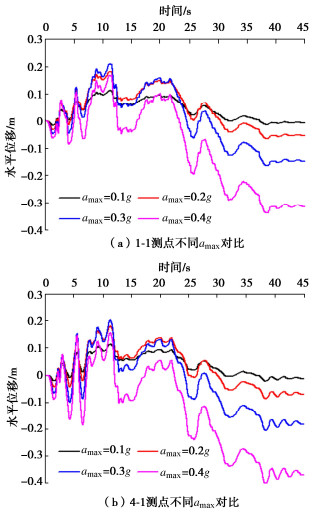
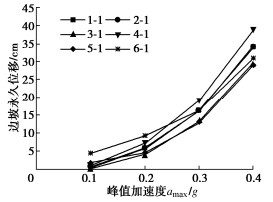
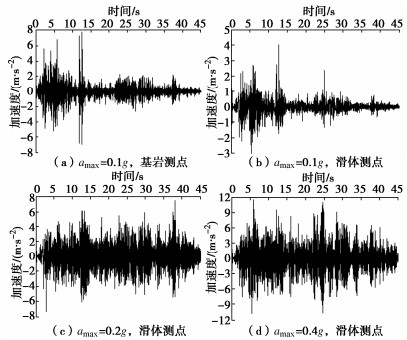
Seismic performance analysis of frame beams-reinforced slope under different earthquake intensities

DAI Xuan^1,,
MA Yunxiang¹,
WEI Shaowei^{2, 3, ,},
WEI Peiyong^{2, 3},
HUO Haifeng¹,
CAI Degou^{2, 3},
LI Zhao¹

1.
College of Transportation Science and Engineering, Civil Aviation University of China, Tianjin 300300, China
2.
Railway Engineering Research Institute, China Academy of Railway Sciences Group Co., Ltd., Beijing 100081, China
3.
Beijing Tieke Special Engineering Technological Development Co., Ltd., Beijing 100081, China

摘要

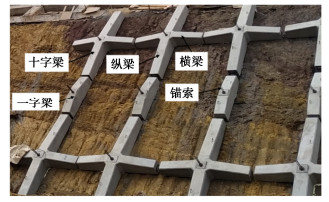
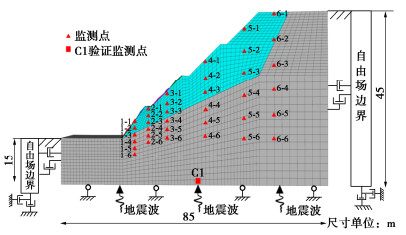
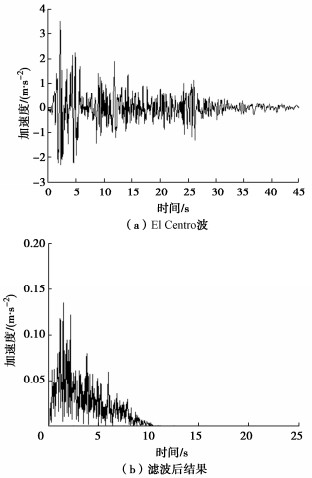
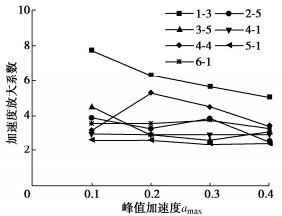
摘要: 装配式预应力锚索框架梁作为一种新型边坡加固方式，其抗震性能目前仍缺乏研究。针对采用“十字梁”与“一字梁”组合加固边坡，以实际工程为背景，建立了非线性动力时程分析数值模型。将El Centro波作为地震动输入，通过调幅分析了不同地震强度的影响规律。研究结果表明，装配式框架梁加固后边坡的滑坡体与基岩水平位移差明显降低，峰值加速度对滑坡体内测点水平位移的影响主要体现在残余变形阶段，边坡的震后永久位移随峰值加速度的增加而增加，且增速逐步加快；在不同峰值加速度下，边坡加速度的响应均出现一定的滞后作用，当峰值加速度较大时，边坡的加速度响应长期处于剧烈波动状态，加固后加速度放大系数最高可达8.0；地震时预制一字梁的梁底应力最大值明显大于预制十字梁，当峰值加速度为0.4g时，一字梁梁底有拉裂风险。
- 装配式锚索框架结构 /
- 边坡加固 /
- 非线性动力分析 /
- 抗震性能
Abstract: As a new slope reinforcement method, the prefabricated prestressed anchor cable frame has been used in engineering. However, its seismic performance still needs to be studied deeply. Based on a practical project, a nonlinear dynamic time history numerical model is established for the reinforcement of slope by the combination of "cross beam" and "straight beam" using El Centro waves as the ground motion input. The influence rules of different earthquake intensities are analyzed through amplitude modulation. The results show that the horizontal displacement difference of the slope after the reinforcement of the frame beams decreases significantly. The influences of the peak acceleration on the horizontal displacement of the slope are mainly reflected at the residual deformation stage. The permanent displacement of the slope after earthquake increases with the increasing peak acceleration, and the growth rate is gradually accelerated. Under different peak accelerations, the slope acceleration response has a certain lag effect. When the peak acceleration is large, the slope acceleration response is in a state of drastic fluctuation for a long time. After reinforcement, the acceleration amplification coefficient can reach 8.0, and gradually decreases with the increase of earthquake intensity. The maximum stress at the bottom of the prefabricated cross beams is obviously smaller than that of the prefabricated straight ones. When the peak acceleration is 0.4g, the bottom of the frame beams has the risk of cracking.
- prefabricated anchor cable frame /
- reinforced slope /
- nonlinear dynamic analysis /
- seismic performance

HTML全文

0. 引言

非饱和状态下的土体具有很高的强度^[1]，然而遇水湿化强度会迅速降低，局部可能达到饱和，该状态下的土压力值与非饱和条件下的值差别很大。多名学者统计显示大部分基坑事故都与水有关，此外，2019年6月8日南宁绿地中心基坑塌陷也是因为场地管道爆裂，非饱和土遇水湿化，作用在支护结构的土压力增大^[2]。因此，亟需定量评估浸湿作用对非饱和土侧向土压力的影响，提出计算方法，减少此类事故发生。

目前，对非饱和土压力研究获得了很大进展，但现有研究多从理论出发进行公式推导，1961年Coleman等^[3]提出双变量理论，Fredlund便得到净应力与吸力的双变量理论，之后得到了扩展的朗肯土压力理论，但是在平时的设计和研究中，仍然采用朗肯土压力理论^[4]计算非饱和土压力。姚攀峰等^[5]提出了与扩展型朗肯土压力不同的计算方法广义朗肯土压力计算方法，陈铁林等^[6]解决水位变化及降水条件下的土压力计算问题，根据K₀定义推导K₀求解式。任传健等^[7]结合Fredlund非饱和土抗剪切与强化准则和经典的朗肯土压计算公式，得出考虑降水变化的土压计算公式。汪丁建等^[8]在饱和土朗肯土压力分析基础上，推导出降雨条件下非饱和朗肯土压力。王晓亮等^[9]将降雨和蒸发对基质吸力的影响引入到非饱和土抗剪强度公式中，得到K₀随降雨定性变化，但没有定量结果。

已有的大量研究充分表明水对静止土压力的影响不可忽略，但已有的计算公式复杂不实用，结果有待验证。导致现有非饱和土体仍采用饱和土理论的计算结果加安全储备来设计计算^[10]，安全系数是否足够不明确。为了使湿化条件下静止土压力增量的演化规律更明确，本文通过室内试验确定了其相关的变化规律、建立相应的计算模型，减小对安全施工的威胁。

1. 试验材料与方法

1.1 土料特性

取北京延庆地区原状粉质黏土进行烘干、碾碎、过0.25 mm筛备用，进行基本物理性质测试，依据《土工试验方法标准：GB/T50123—2019》^[11]，结果见表 1。

表 1 土的基本物理性质

Table 1. Basic physical properties of soil

最大干密度/ (g·cm^-3)	最优含水率/%	液限 w_L/%	塑限w_P/%	塑性指数I_P	土粒相对密度G_S
1.80	16.5	30.7	15.2	15.5	2.73

下载: 导出CSV

| 显示表格

1.2 试验方案

选择干密度1.53 g/cm³（压实度0.85）、高度40 mm的标准环刀试样开展K₀压缩试验，设5个不同的初始饱和度与4个不同的上覆荷载，具体方案见表 2。

表 2 浸水条件下非饱和粉质黏土试验方案

Table 2. Test schemes under water immersion conditions

上覆荷载/kPa	加载过程	初始饱和度
100/200/ 300/400	100(200/300/400)kPa→湿化→逐级加载至1600kPa	0.2/0.3/0.4/ 0.5/0.6

下载: 导出CSV

| 显示表格

1.3 试验过程

（1）仪器标定。本文采用JCY型K₀固结仪来完成K₀压缩试验，在气囊中充入与试样等体积的水，利用水各向等压特性标定仪器在竖向压力下对土压力的测量，根据试验数据拟合得到两仪器的标定系数^[12]。

（2）制样并养护得到不同初始含水率试样。用饱和再风干的土样模拟经过了干湿循环的天然非饱和土，通过7 d密闭养护保证孔隙水分布均匀，见图 1。

图 1 准备不同初始含水率的试样

Figure 1. Preparation of samples with different initial moisture contents

下载: 全尺寸图片幻灯片

（3）加上覆荷载待稳定后进行湿化饱和，湿化稳定后养护7 d，再完成后续设定加载至试验结束。

（4）卸压并整理仪器装置，将不同初始饱和度湿化前与湿化压缩后试样进行对比，如图 2所示。

图 2 试验前后对比图

Figure 2. Comparison of soil samples before and after tests

下载: 全尺寸图片幻灯片

2. 试验结果及规律

K₀固结仪连接压力传感器采集数据，得到侧压力随时间变化关系^[12]，从而得到粉质黏土在5个不同初始饱和度S_r和4个不同上覆荷载P作用下发生湿化与湿化后继续加载的水平静止土压力-竖向压力的关系曲线，见图 3，因篇幅关系只展示S_r=0.2结果^[12]。对于非饱和土一般采用水土合算计算土压力，此时侧压力传感器测量得到的相当于水土合算下的土压力。

图 3 静止土压力随竖向压力变化关系(S_r=0.2)

Figure 3. Variation of static earth pressure with vertical pressure (S_r=0.2)

下载: 全尺寸图片幻灯片

湿化静止土压力增量 $\Delta {\sigma _{{\text{h}}}}$ 统计见表 3，计算式为

$\Delta {\sigma _{{\text{h}}}} = {\sigma _{{\text{w}}}} - {\sigma _{{\text{d}}}} 。$

(1)

表 3 湿化静止土压力增量计算值统计

Table 3. Statistics of calculated increment static earth pressure

初始饱和度S_r	0.2	0.3	0.4	0.5	0.6
100 kPa下增量值	35.14	25.10	17.41	12.5	3.53
200 kPa下增量值	68.95	48.38	33.32	22.97	6.31
300 kPa下增量值	95.01	68.95	47.86	29.99	8.98
400 kPa下增量值	118.02	90.00	60.99	35.97	10.11

下载: 导出CSV

| 显示表格

式中： ${\sigma _{{\text{d}}}}$ 为上覆荷载作用下湿化前静止土压力大小； ${\sigma _{{\text{w}}}}$ 湿化饱和后静止土压力大小。

3. 静止土压力增量计算模型

3.1 湿化过程中静止土压力演变规律

不同初始饱和度湿化过程的增湿水平不同，可使用湿化前初始饱和度表示增湿水平，即：S_r=1的增湿水平为0，S_r越小增湿水平越大。

由表 3可以看到湿化时静止土压力都有不同程度的增大，且初始饱和度S_r越低或上覆荷载P越大，静止土压力增量越大。图 3数据显示，湿化后继续加载呈线性且斜率基本一致，表明K₀值大小近似一致，S_r与P的不同不会影响湿化饱和后K₀大小。可能原因是：静止土压力系数主要由有效内摩擦角决定，饱和后有效内摩擦角接近，因此湿化饱和后K₀近似一致。

土体强度理论认为土颗粒间存在综合作用，包括吸力、胶结作用、德华力以及化学键等^[4]，非饱和土研究学者^[13]一般认为土骨架受压为保证完整性依靠两部分力平衡：一是土颗粒间的基质吸力，取决于土体的含水量；另外是土颗粒间的胶结力，取决于土体内部的黏粒微量物质。静止土压力增量是由颗粒间胶结作用的减弱和基质吸力减小两方面原因引起的^[14]。为推导计算模型引出中间变量0.65-S_r，如图 4所示，初始饱和度越小，湿化导致基质吸力减少量就越大，静止土压力增量就越大；湿化饱和后上覆荷载越大，对土体胶结力破坏就越大，如图 5所示，湿化饱和后的静止土压力增量，随上覆荷载增加而变大。

图 4 静止土压力增量与初始饱和度关系

Figure 4. Variation of increment of static earth pressure increment with initial saturation

下载: 全尺寸图片幻灯片

图 5 静止土压力增量与上覆荷载关系

Figure 5. Variation of increment of static earth pressure with load

下载: 全尺寸图片幻灯片

3.2 湿化过程中静止土压力增量计算模型

土压力增量 $\Delta {\sigma _{{\text{h}}}}$ 与上覆荷载P，初始饱和度S_r都呈线性关系，双线性模型见式（2），P和S_r确定时有一次函数式（3），（4）。当变量 $n=（{S}_{\text{r}}{\text{+}}{b}_{1}）{\text{=0}}{\text{.65}}-{S}_{{\text{r}}}$ 时，土压力增量 $\Delta {\sigma _{{\text{h}}}}$ 与n成正比例， ${k_1}{k_2}m$ 为斜率，见图 4。

$\Delta {\sigma _{{\text{h}}}} = {k_1}n \times {k_2}m {\text{，}}$

(2)

${k}_{1}n={k}_{1}（{S}_{{\text{r}}}{\text{+}}{b}_{1}） {\text{，}}$

(3)

${k}_{2}m={k}_{2}（P{\text{+}}{b}_{2}）。$

(4)

P与其对应的 ${k_1}{k_2}m$ 拟合得 ${k_1}{k_2}m{{\text{ = 0}}}{{\text{.60}}}P + {{\text{19}}}{{\text{.76}}}$ ，再将 $n$ 代入式（2）中，得到式（5）。当初始饱和度S_r较大接近饱和土时，静止土压力增量为0，观察式（5），当饱和度S_r＞0.65时，湿化不会引起静止土压力增加。

$\Delta {\sigma _{{\text{h}}}} = \left\{ \begin{array}{l} (0.60P{{\text{ + 19}}}{{\text{.76}}}){{\text{(}}}0.65 - {S_{{\text{r}}}}{{\text{)}}} \;\;\;\;{{\text{(}}}{S_{{\text{r}}}} \leqslant 0.65{{\text{)}}} \hfill \\ 0\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;{{\text{ (}}}{S_{{\text{r}}}} > 0.65{{\text{)}}} \hfill \\ \end{array} \right. 。$

(5)

为了更直观的表现增量的含义，将 $\Delta {S_{{\text{r}}}} = 1 - {S_{{\text{r}}}}$ 代入式（5），得到最终的增量表达式如下：

$\begin{equation} \Delta \sigma_{\mathrm{h}}=\left\{\begin{array}{ll} (0.60 P+19.76)\left(\Delta S_{\mathrm{r}}-0.35\right) & \left(\Delta S_{\mathrm{r}} \geqslant 0.35\right) \\ 0 & \left(\Delta S_{\mathrm{r}}<0.35\right) \end{array}\right. 。 \end{equation}$

(6)

4. 挡土墙静止土压力计算案例分析

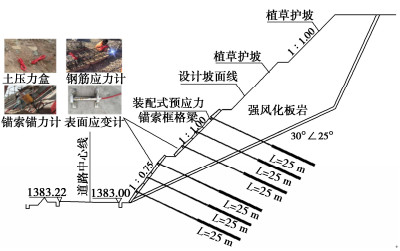
以延庆某深基坑为背景，结合勘察数据，对上文的模型进行试算。该基坑开挖深度23 m，上表面有8 kPa的均布荷载，施工阶段饱和度0.25，已勘测到自然地面以下34 m地层特性，土体基本为粉质黏土。

根据划分土层的重度与厚度计算出土层下表面荷载，并根据K₀算出湿化前静止土压力 ${\sigma _{{\text{h}}}}_i$ ，K₀按经验值取0.3。根据式（6）算出静止土压力增量 $\Delta {\sigma _{{\text{h}}}}_i$ ，接着计算出湿化后静止土压力 ${\sigma _{{\text{w}}}}_i$ 和 ${\sigma _{{\text{w}}}}_i$ / ${\sigma _{{\text{h}}}}_i$ 比值，计算值随深度变化绘制在图 6中，发现比值随深度增大而减小，但始终大于1.8，说明湿化对静止土压力影响较大。

图 6 不同累计深度处静止土压力与其相关计算值关系

Figure 6. Static earth pressures and their correlation with depth

下载: 全尺寸图片幻灯片

由于本文采用重塑土进行试验，和天然土体湿化时侧压力变化结果不同，特别是黄土等结构性非饱和土，其湿化可能发生湿陷等行为，导致土压力演化较为复杂。本文研究结果仅适用于非结构性的非饱和土。

5. 结论

本文通过开展室内试验，定量评估浸湿作用对非饱和土侧向土压力的影响，实测浸湿饱和作用下静止土压力增量的变化规律，建立相应的计算模型，通过应用发现设计时必须重视湿化的影响，并得到以下3点结论。

（1）湿化饱和后，土体的静止土压力系数K₀值与初始饱和度、上覆荷载无关。推测土体静止土压力系数K₀值主要由有效内摩擦角决定，饱和后有效内摩擦角基本一致，故K₀值大小近似一致。

（2）湿化前的初始饱和度越低，湿化饱和后的静止土压力增量越大；且湿化饱和后的静止土压力增量，随湿化时的上覆荷载增加而变大。

（3）基于试验数据和机理分析，得到了湿化条件下考虑上覆荷载与初始饱和度的双线性土压力增量计算模型；将其应用于某支挡工程，发现湿化后的土压力可达初始土压力1.8倍以上，设计时必须予以重视。

图 1 新型装配式锚索框架梁现场试验

Figure 1. Site photos of prefabricated beams

下载: 全尺寸图片幻灯片

图 2 边坡地质剖面

Figure 2. Geological section of slope

下载: 全尺寸图片幻灯片

图 3 有限差分数值分析模型及其测点分布

Figure 3. FLAC model and monitoring points

下载: 全尺寸图片幻灯片

图 4 输入地震动及其处理

Figure 4. Input seismic waves and filting

下载: 全尺寸图片幻灯片

图 5 预制梁加固边坡效果对比分析（单位：m）

Figure 5. Comparison of reinforcement effects using prefabricated beam on slope deformation

下载: 全尺寸图片幻灯片

图 6 不同峰值加速度下滑坡体内测点水平位移时程曲线对比

Figure 6. Comparison of time-history curves of horizontal displacement at measuring points in landslide under different peak accelerations

下载: 全尺寸图片幻灯片

图 7 不同峰值加速度对边坡永久位移的影响

Figure 7. Influences of different peak accelerations on permanent displacement of slope

下载: 全尺寸图片幻灯片

图 8 不同峰值加速度下加速度时程曲线对比

Figure 8. Comparison of time-history curves of acceleration under different peak accelerations

下载: 全尺寸图片幻灯片

图 9 不同峰值加速度下加速度放大效应

Figure 9. Amplification effects of acceleration under different peak accelerations

下载: 全尺寸图片幻灯片

图 10 框架梁梁底应力时程曲线对比

Figure 10. Comparison of tensile stress-time curves at bottom of beam

下载: 全尺寸图片幻灯片

图 11 不同峰值加速度下框架梁底最大应力对比

Figure 11. Comparison of maximum tensile stresses at bottom of frame beam under different peak accelerations

下载: 全尺寸图片幻灯片

表 1 模型中岩体与框架结构的物理力学参数

Table 1 Parameters of mechanical property used in numerical model

材料	重度γ/(kN·m^-³)	弹性模量/MPa	泊松比	黏聚力c/kPa	内摩擦角φ/(°)
强风化板岩	27.8	80	0.3	60	28
滑面	—	2	0.3	45	23
预制梁	25.0	34175	0.2	—	—

下载: 导出CSV

表 2 预应力锚索数值模拟参数

Table 2 Simulation parameters of prestressed anchor cable

参数	取值	参数	取值
等效锚索直径/m	2.67×10^-2	水泥浆剪切刚度/Pa	3.28×10⁹
横截面积/m²	5.6×10^-4	水泥浆黏结强度/(N·m^-1）	1.47×10⁶
锚索弹性模量/(N·m^-2)	2.00×10¹¹	注浆孔直径/m	0.15
屈服强度/N	9.16×10⁵	水泥浆外圈周长/m	0.471

下载: 导出CSV

参考文献(16)

[1]	ZHANG J J, NIU J Y, FU X, et al. Failure modes of slope stabilized by frame beam with prestressed anchors[J]. European Journal of Environmental and Civil Engineering, 2022, 26(6): 2120-2142. doi: 10.1080/19648189.2020.1752806
[2]	ZHANG J H, ZHOU Q W, LI F, et al. Case study of field application of prefabricated anchoring frame beam structure in slope supporting projects[J]. Journal of Construction Engineering and Management, 2022, 148(9): 50208. http://www.nstl.gov.cn/paper_detail.html?id=c8c2f5045fa0da1601e66989f16fea78
[3]	刘华. 预制混凝土格构锚固体系的设计方法研究[D]. 成都: 西南交通大学, 2015. LIU Hua. Study on the PC Frame Anchor System and the Design Method Research[D]. Chengdu: Southwest Jiaotong University, 2015. (in Chinese)
[4]	CHEN Z Y, WANG Z, XI H, et al. Recent advances in high slope reinforcement in China: case studies[J]. Journal of Rock Mechanics and Geotechnical Engineering, 2016, 8(6): 775-788. doi: 10.1016/j.jrmge.2016.11.001
[5]	ZHU H W, XIANG Q, LUO B, et al. Evaluation of failure risk for prestressed anchor cables based on the AHP-ideal point method: an engineering application[J]. Engineering Failure Analysis, 2022, 138: 106293. doi: 10.1016/j.engfailanal.2022.106293
[6]	YIN J H. Comparative modeling study of reinforced beam on elastic foundation[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2000, 126(3): 265-271. doi: 10.1061/(ASCE)1090-0241(2000)126:3(265)
[7]	张军辉, 周勤伟, 黎峰, 等. 框架梁锚固边坡支护结构研究进展综述[J]. 中外公路, 2023, 43(3): 17-23. https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL202303002.htm ZHANG Junhui, ZHOU Qinwei, LI Feng, et al. Review of research progress of frame beam anchored structure in slope supporting[J]. Journal of China & Foreign Highway, 2023, 43(3): 17-23. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GWGL202303002.htm
[8]	董建华, 董旭光, 朱彦鹏. 随机地震作用下框架锚杆锚固边坡稳定性可靠度分析[J]. 中国公路学报, 2017, 30(2): 41-47. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201702006.htm DONG Jianhua, DONG Xuguang, ZHU Yanpeng. Reliability analysis on stability of slope reinforced by frame with pre-stress anchors under random earthquake action[J]. China Journal of Highway and Transport, 2017, 30(2): 41-47. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZGGL201702006.htm
[9]	DENG D P, ZHAO L H, LI L. Limit-equilibrium analysis on stability of a reinforced slope with a grid beam anchored by cables[J]. International Journal of Geomechanics, 2017, 17(9): 6017011-6017013. doi: 10.1061/(ASCE)GM.1943-5622.0000929
[10]	NIU J Y, ZHANG J J, CHEN F Y, et al. Experimental and numerical investigation on the seismic response of slope reinforced by frame beam and anchor cable[J]. Arabian Journal of Geosciences, 2022, 15(5): 1-19.
[11]	魏少伟, 包秀明, 姚建平, 等. 基于铁木辛柯梁理论解的装配式预应力锚索框架梁受力特征分析[J]. 铁道建筑, 2021, 61(11): 78-81. https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202111017.htm WEI Shaowei, BAO Xiuming, YAO Jianping, et al. Mechanical characteristics analysis of prefabricated prestressed-anchor-cable frame-beam based on timoshenko-beam theoretical solution[J]. Railway Engineering, 2021, 61(11): 78-81. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202111017.htm
[12]	魏少伟, 蔡德钩, 姚建平, 等. 装配式预应力锚索框架结构设计与施工关键技术[J]. 铁道建筑, 2022, 62(2): 15-24. https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202202004.htm WEI Shaowei, CAI Degou, YAO Jianping, et al. Design and construction key technology of prefabricated prestressed anchor cable frame structure[J]. Railway Engineering, 2022, 62(2): 15-24. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202202004.htm
[13]	CAO L C, ZHANG J J, WANG Z J, et al. Dynamic response and dynamic failure mode of the slope subjected to earthquake and rainfall[J]. Landslides, 2019, 16(8): 1467-1482. doi: 10.1007/s10346-019-01179-7
[14]	YE S H, FANG G W, ZHU Y P. Model establishment and response analysis of slope reinforced by frame with prestressed anchors under seismic considering the prestress[J]. Soil Dynamics and Earthquake Engineering, 2019, 122: 228-234. doi: 10.1016/j.soildyn.2019.03.034
[15]	王新红, 秦川, 魏培勇, 等. 装配式锚索框架在边坡支护工程中的应用[J]. 铁道建筑, 2023, 63(2): 134-138. https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202302026.htm WANG Xinhong, QIN Chuan, WEI Peiyong, et al. Application of fabricated anchor cable frame in slope support engineering[J]. Railway Engineering, 2023, 63(2): 134-138. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDJZ202302026.htm
[16]	工程岩体分级标准: GB/T 50218—2014[S]. 北京: 中国计划出版社, 2015. Standard for Engineering Classification of Rock Mass: GB/T 50218—2014[S]. Beijing: China Planning Press, 2015. (in Chinese)