吹填淤泥真空预压固结机理与排水体防淤堵处理技术

蔡袁强

doi:10.11779/CJGE202102001

吹填淤泥真空预压固结机理与排水体防淤堵处理技术 English Version

蔡袁强

浙江工业大学土木工程学院,浙江　杭州　310014

详细信息

作者简介:
蔡袁强(1965— )，男，博士，教授，主要从事地基处理、基础工程学、土动力学等方面的教学和科研工作。E-mail：caiyq@zju.edu.cn

中图分类号: TU43
计量
- 文章访问数: 712
- HTML全文浏览量: 27
- PDF下载量: 761
出版历程
- 收稿日期: 2020-12-24
- 网络出版日期: 2022-12-04
- 刊出日期: 2021-01-31

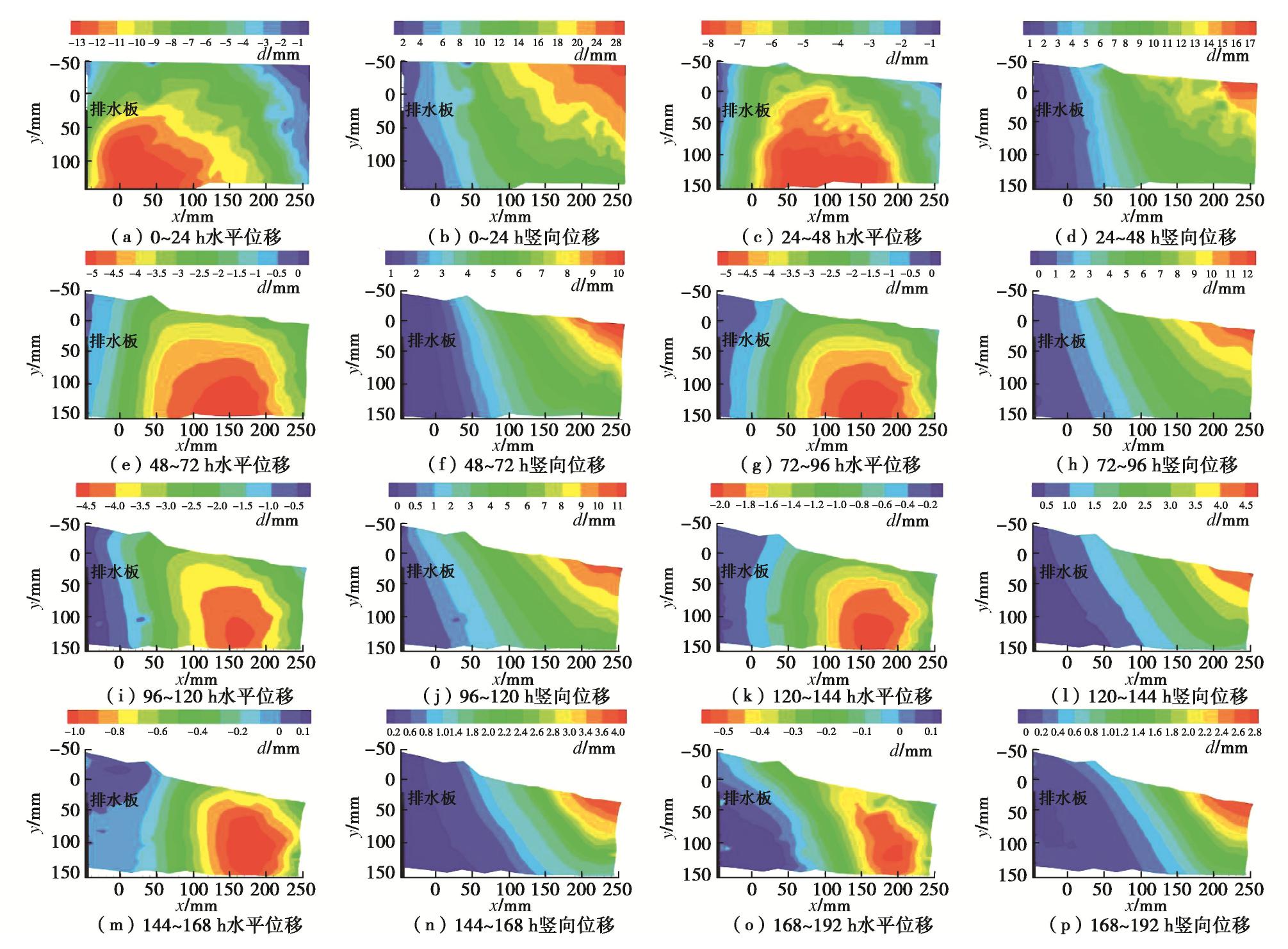
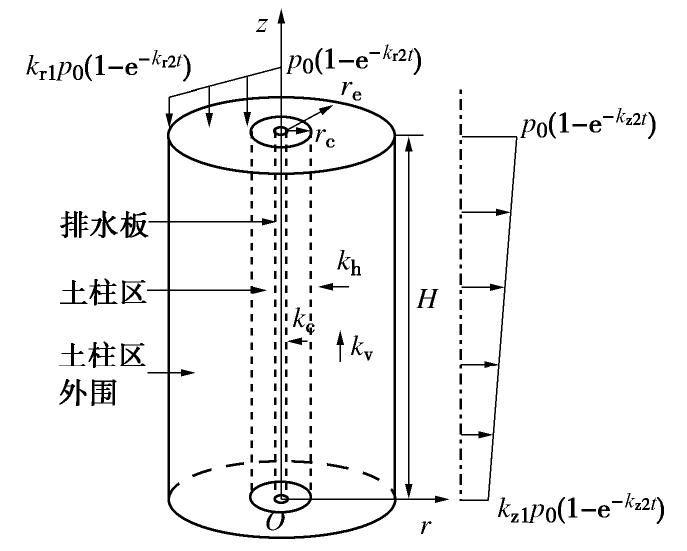
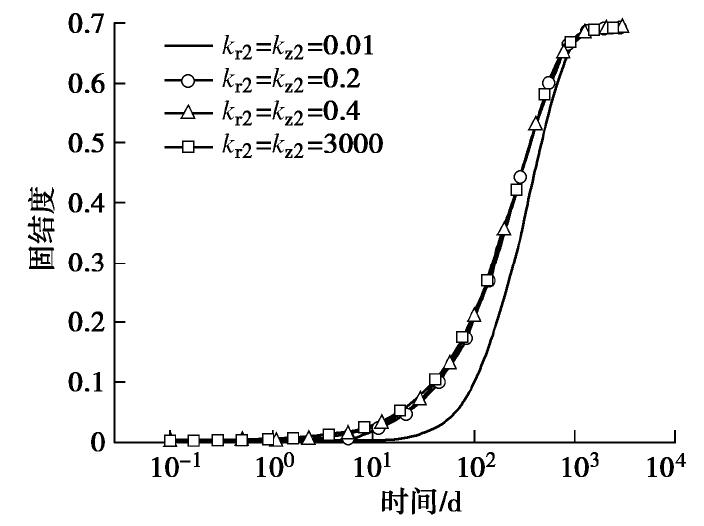
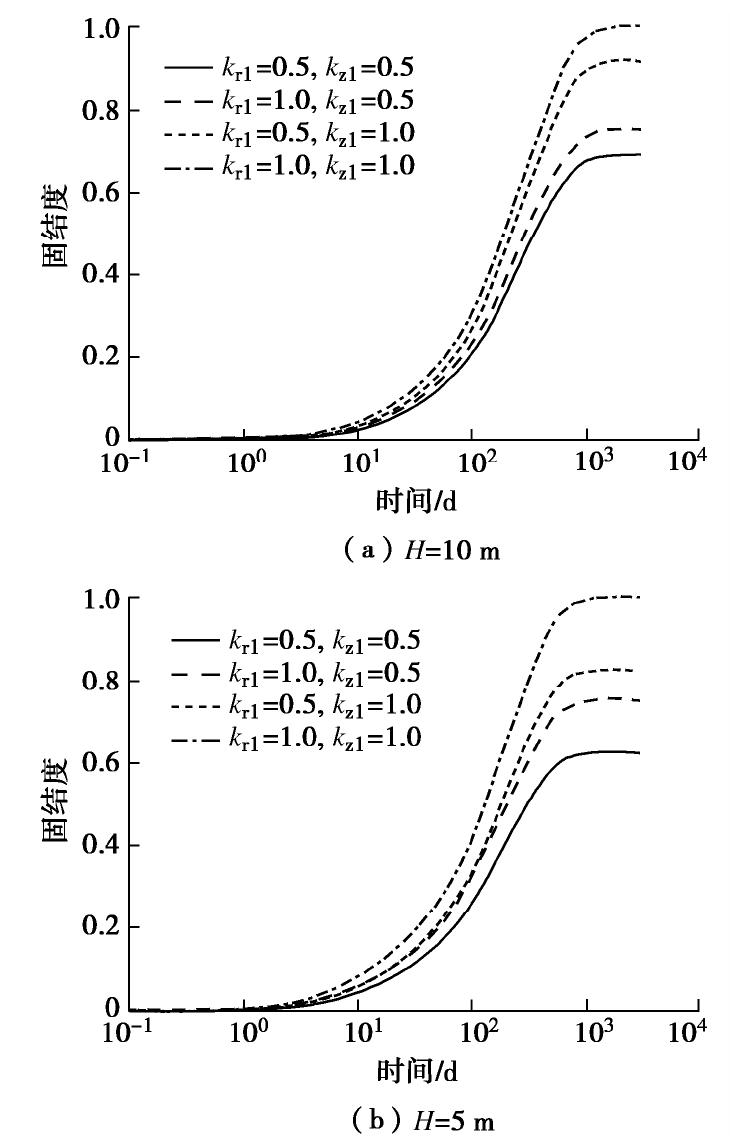
Consolidation mechanism of vacuum preloading for dredged slurry and anti-clogging method for drains

CAI Yuan-qiang

College of Civil Engineering, Zhejiang University of Technology, Hangzhou 310014, China

摘要

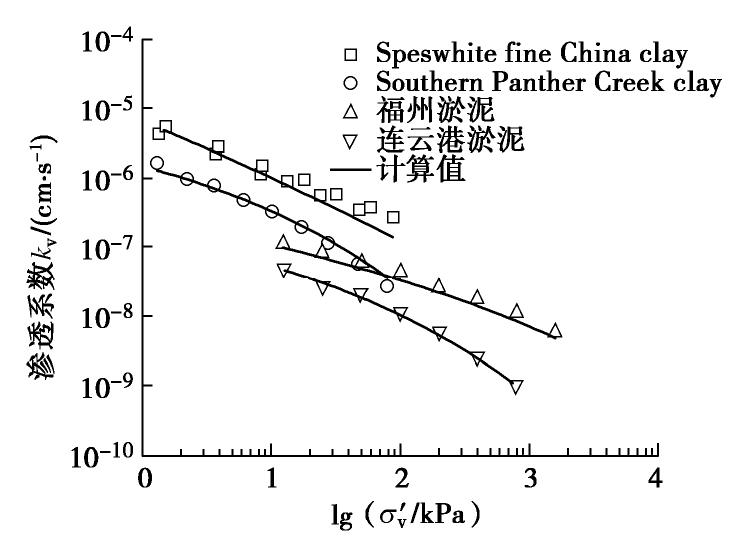
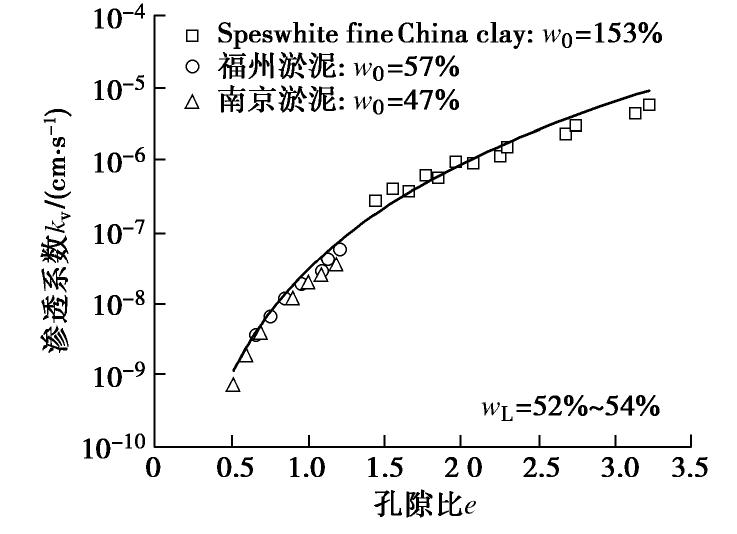
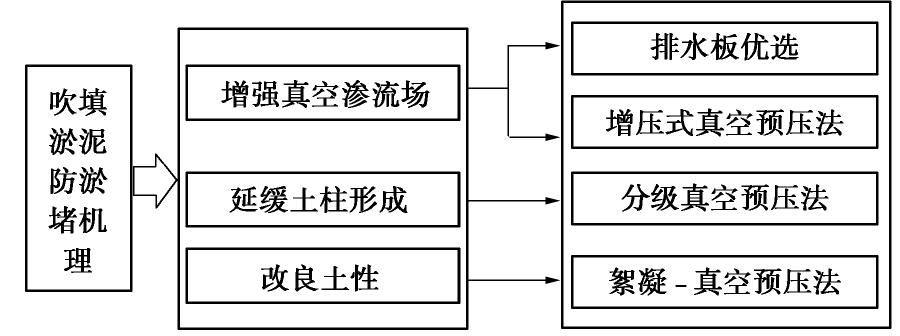
摘要: 针对吹填淤泥真空预压地基处理效果差的关键问题,全面总结了笔者及其课题组多年来的研究成果,主要包括4个方面：①采用超低压固结-渗透仪进行了大量的吹填淤泥固结、渗透试验,发现了初始含水率和液限对压缩-渗透性状的影响规律,建立了压缩过程中渗透系数的定量演化规律。②吹填淤泥真空预压过程中的位移、应变发展规律和土柱形成机理。基于粒子图像测速（PIV）技术和粒子追踪测速（PTV）技术,获得了吹填淤泥真空预压固结过程中的土体位移、应变发展规律,揭示了吹填淤泥真空预压固结机理,获得了真空预压作用下不同初始含水率和不同级配吹填淤泥的土柱形成过程。③吹填淤泥真空预压固结计算方法。基于不均匀应变假定提出了土柱区内、外固结计算公式,获得了不同边界真空度时空变化下的固结规律。以土柱形成过程为基础,建立了考虑土柱影响的吹填淤泥真空预压固结计算方法。④通过室内和现场试验,从增强真空渗流场、延缓土柱形成和改良土性等方面,优化排水板滤膜孔径,提出了分级、增压式、絮凝-真空预压等多项防淤堵处理技术,缓解排水体淤堵,提高固结速率,加速吹填淤泥处理,增强加固效果。研究成果对吹填淤泥场地开发利用,缓解城市土地资源紧张,促进城市可持续发展具有重要意义。
- 吹填淤泥 /
- 真空预压 /
- 固结 /
- 土柱 /
- 增压 /
- 絮凝
Abstract: Considering the key problem that the vacuum preloading method cannot efficiently improve dredged slurry, the research findings over the years obtained by the author’s research team are comprehensively summarized, including the following four aspects: (1) A large number of consolidation-hydraulic conductivity tests are performed using the modified consolidation apparatus under low initial stress. Based on the test results, the influences of the initial water content and the liquid limit on the compression-permeability properties are discussed. The evolution laws of the permeability coefficient during the compression are obtained quantitatively. (2) The development laws of displacement and strain of soils and the formation mechanism of soil column during the vacuum preloading consolidation of the dredged slurry are illustrated. By utilizing the particle image velocimetry (PIV) and the particle tracking velocimetry (PTV) techniques, the development laws of the displacement and strain of soils during the vacuum preloading consolidation process are obtained. Furthermore, the consolidation process of the dredged slurry is revealed. Besides, the formation process of the soil columns under different initial water contents and particle-size distributions is obtained. (3) The analytical and numerical methods are proposed to calculate the consolidation of dredged slurries improved by vacuum preloading. Based on the uneven strain assumption, the consolidation solutions are derived for the clogging zone and the intact zone, respectively. Subsequently, the consolidation behaviors are studied under various distributions and development laws of vacuum preloading at the permeable boundaries. Besides, the consolidation model considering the formation of the soil columns is also established to investigate the relevant influences. (4) Based on the laboratory and field tests, several methods are proposed by enhancing the vacuum seepage fields, slowing the formation of soil columns, and improving the soil properties, to increase the consolidation efficiency. These methods include applying staged vacuum preloading, booster PVDs, multi-flocculant treatment, and appropriate pore size of PVD filters. Using these methods, the clogging of the dredged slurry during the treatment process can be reduced, and then the consolidation speed and improvement quality can be increased. The research findings are of great significance to developing and utilizing dredging slurry sites, alleviating the shortage of urban land resources, and promoting the sustainable urban development.
- dredged slurry /
- vacuum preloading /
- consolidation /
- clogging /
- vacuum gradient /
- flocculation

HTML全文

0. 引言

持水特征曲线指土体持水状态（可用体积含水率、质量含水率或饱和度表达）与土中水的能量状态（可用吸力、势能或相对湿度表达）之间的关系^[1],描述了土中水（液相）与土（固相）间的能量平衡状态^[2]。

土体持水的物理化学机制有毛细、吸附与渗透。毛细机制与弯液面上的表面张力有关,受Young- Laplace方程控制（土体孔径越小其毛细吸力越大）,当土中水能量状态低至其空化点以下,毛细水汽化,相应毛细机制消失。吸附机制指（黏）土颗粒表面由于van der Waals力、双电层力、阳离子水化等造成的短程吸附作用导致的土中水能量降低,可用Kelvin方程描述。通常将毛细与吸附机制导致的土中水相对自由水状态而言势能的降低称为基质势（用负值表达）；将对应土体固相的持水/吸水能力（用正值表达）称为基质吸力；二者数值相等,符号相反。此外,渗透机制指溶解盐会降低土中水的能量状态,其能量降低程度与溶质类型和浓度相关。

SWRC表征土体持水能力,原本用于农业领域,随后的研究表明非饱和状态下的渗流、强度与变形等土力学行为^[1]乃至土的成分与结构等土质学特征^[2]均与SWRC密切相关,因而成为描述非饱和状态下土体性状的关键指标与工具,在非饱和土力学研究与工程应用中具有基础的、特殊的、关键的作用与位置,其研究成果亦非常丰富^[1-3],但大部分SWRC^[1-3]由轴平移方法获得,受陶土板进气值限制,其基质吸力目前仅能控制在1500 kPa以内,根据Kelvin方程（式（1））可知其对应的相对湿度RH为98.9%以上（环境温度25℃时）。然而,自然界中RH变化范围远比轴平移方法所能控制的RH范围大；例如中国主要城市各月RH统计^[4]显示,最低14%（拉萨,2月）,最高90%（长沙,10月与11月；海口,1月）；因而高吸力（RH低于95%或吸力高于7.1 MPa）下的土体持水特征研究具有特别的现实意义。

高吸力下土体持水特征测试常用方法主要有：滤纸法^[5-8]（适用于10～100 MPa）、冷镜湿度计法^[9]（适用于0～300 MPa）、饱和盐溶液等压湿度控制法^[10-12]（适用于3～400 MPa）、干湿空气混合湿度控制法^[13]（适用于7～600 MPa）等。前两种为吸力量测方法,难以控制吸力；后两种为吸力控制方法,适于测定完整的SWRC,但蒸汽平衡历时漫长,如文献[14]采用饱和盐溶液等压湿度控制法测定3.3～359.1 MPa吸力范围的膨胀土SWRC耗时319 d,阻碍了高吸力下土体持水特征的研究与应用。

近年来出现的动态露点等温线方法^[15-16]使得上述情况有所改观,该方法能够在较短时间（几天内）获得相对湿度在3%～95%范围的SWRC。为了给高吸力下相关土力学工程实践提供依据和参考,本文采用该方法：①对从黏土质砂到极高塑性黏土的9种土样开展高吸力下吸脱湿过程持水特征测试；②采用RH = 95%下的吸湿质量含水率w₉₅量化高吸力下持水能力,采用吸脱湿SWRC间滞回区域面积HHA量化高吸力下SWRC滞回效应强弱,采用同一吸力下吸脱湿SWRC含水率之差最大值Δw_max量化水力滞回导致的含水率偏差范围；③探讨w₉₅、HHA、Δw_max与液限w_L、塑性指数I_p、阳离子交换量CEC、比表面积SSA等土性参数间量化关系；④构建修正的Fredlund-Xing模型描述高吸力下吸脱湿过程SWRC。

1. 研究方案

1.1 试验土样

试验采用Wyoming膨润土、宁明膨胀土、荆门黄褐色膨胀土、Denver黏土岩、荆门棕褐色膨胀土、武汉黏土、三门峡粉质黏土、郑州粉土和开封黄河砂共9种土样,其物理性质指标测试结果见表1。

表 1 试验用土的物理性质指标

Table 1. Physical property indexes of soils

土样名称	颗粒相对质量密度^[17]	颗粒所占比例^[17]/%				液限^[17]/%	塑限^[17]/%	塑性指数	塑性^[18]	USCS 定名^[19]	比表面积EGME^[20]/(m²·g^-1)	CEC(NH₄⁺)^[17]/( mmol·kg^-1)	自由膨胀率^[21]/%	膨胀潜势^[21]
土样名称	颗粒相对质量密度^[17]	>0.075 mm	0.005~0.075 mm	0.002~0.005 mm	<0.002 mm	液限^[17]/%	塑限^[17]/%	塑性指数	塑性^[18]	USCS 定名^[19]	比表面积EGME^[20]/(m²·g^-1)	CEC(NH₄⁺)^[17]/( mmol·kg^-1)	自由膨胀率^[21]/%	膨胀潜势^[21]
Wyoming膨润土	2.70	5.1	14.2	3.8	77.0	217.7	33.1	184.5	极高	CH	500.1	621	775	强
宁明膨胀土	2.73	5.8	2.9	0.3	91.1	164.6	37.6	127.0	极高	CH	393.0	508	655	强
荆门黄褐色膨胀土	2.75	2.1	47.4	21.5	29.0	62.9	25.5	37.4	高	CH	236.5	309	75	中
Denver黏土岩	2.72	8.5	44.2	20.6	26.7	46.0	23.2	22.8	中等	CL	88.7	160	38	—
荆门棕褐色膨胀土	2.72	11.6	42.4	26.7	19.3	41.8	20.7	21.1	中等	CL	110.4	254	40	弱
武汉黏土	2.73	5.5	63.9	9.6	21.0	40.3	18.2	22.1	中等	CL	112.5	154	39	—
三门峡粉质黏土	2.72	0.5	82.8	5.7	11.1	35.2	19.1	16.1	中等	CL	108.5	149	30	—
郑州粉土	2.70	8.7	77.4	2.5	11.4	26.1	14.8	11.3	低	CL	27.7	50	9	—
开封黄河砂	2.68	62.6	36.0	0.2	1.3	—	—	—	—	SC	7.5	16	—	—

下载: 导出CSV

| 显示表格

1.2 蒸汽吸附分析仪工作原理

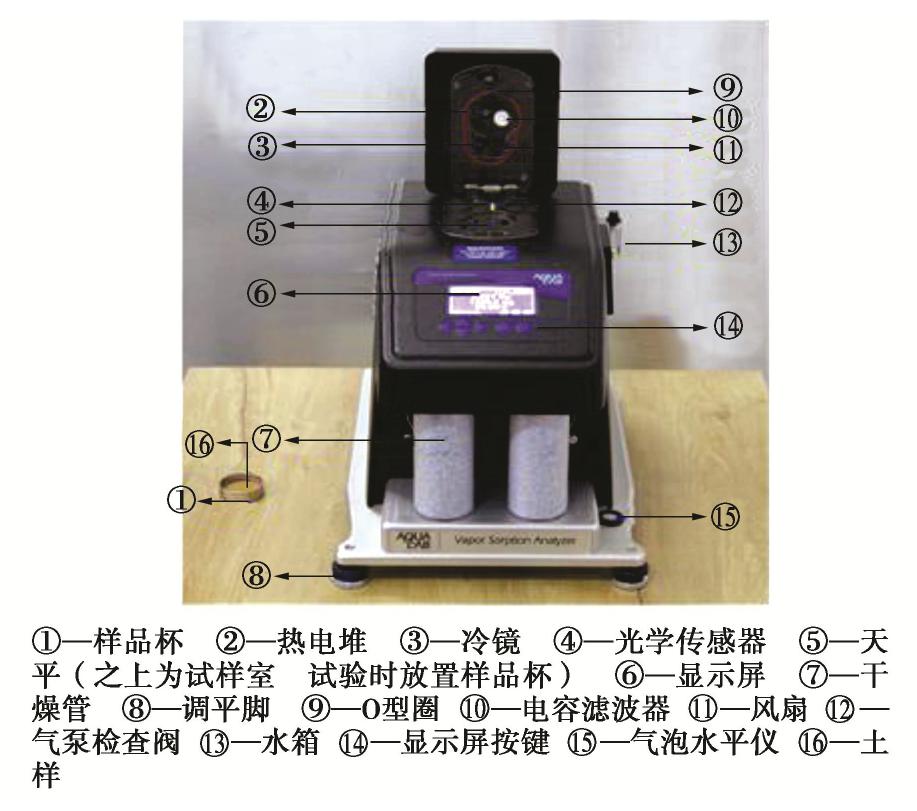
美国Meter公司生产的蒸汽吸附分析仪（Vapor Sorption Analyzer, VSA）见图1,用于测试动态的或平衡的水分吸附等温线（moisture sorption isotherms）,即给定温度下的SWRC。

图 1 蒸汽吸附分析仪

Figure 1. Vapor sorption analyzer

下载: 全尺寸图片幻灯片

VSA采用动态露点等温线（dynamic dew point isotherm, DDI）方法获得动态水分吸附等温线；采用控制湿度下测试样质量的方法（dynamic vapor sorption, DVS）获得静态（平衡）水分吸附等温线。其工作原理是：DDI方法既不控制含水率亦不控制RH,而是在用湿气流湿化/干气流脱湿过程中通过高精度天平测试样质量变化、通过冷镜露点法测RH得到动态水分吸附等温线。DVS方法是在控制RH条件下跟踪试样质量变化以获得静态（平衡）水分吸附等温线,每级湿度下的稳定（平衡）标准可用预设时间间隔或预设试样质量变化幅度。Likos等^[15]与Arthur等^[22]试验证实了DDI方法的精度与可靠性,系统试验研究表明DDI方法与DVS方法获得的SWRC无明显偏差。

VSA相对湿度控制范围是3%～95%（25℃下对应吸力范围为7.1～482.9 MPa）,试样质量建议范围为500～5000 mg,试样温度可控范围为15℃～60 ℃。

1.3 高吸力下持水特征试验方案

采用VSA测定9种土样高吸力下SWRC。将土样风干碾散过0.5 mm筛,置于烘箱中烘至衡量（105 ℃下烘干48 h）,取出置于干燥器内冷却至室温,每次取约1000 mg试验。试验方案与步骤如下：

（1）仪器准备

将VSA在工作台上调平,安装干燥管,水箱中注入去离子水,检查天平,标定相对湿度。

（2）设定SWRC量测步骤

鉴于采用DDI方法与DVS方法测土样SWRC具有很好的一致性^[22],且前者测试历时约为后者的1/25^[15],因此采用DDI方法。由于试样是完全烘干后的,质量含水率w为0,第1步设置初始RH为3%,最终RH为95%,用DDI方法采集吸湿过程w数据RH,即土样SWRC吸湿边界线；第2步设置初始RH为95%、最终RH为3%,目的是获得土样SWRC的脱湿曲线；由于脱湿曲线起始点为RH=95%对应点,所以并非脱湿边界线。此外,设定样品室温度为25℃。

（3）测定SWRC

将试样置入样品杯,放入试样室开始测试,测试由VSA自动执行。试验结束后,取出试样杯称试样质量,烘干后复测干土质量,并在计算机终端下载SWRC试验数据。

2. 试验结果分析

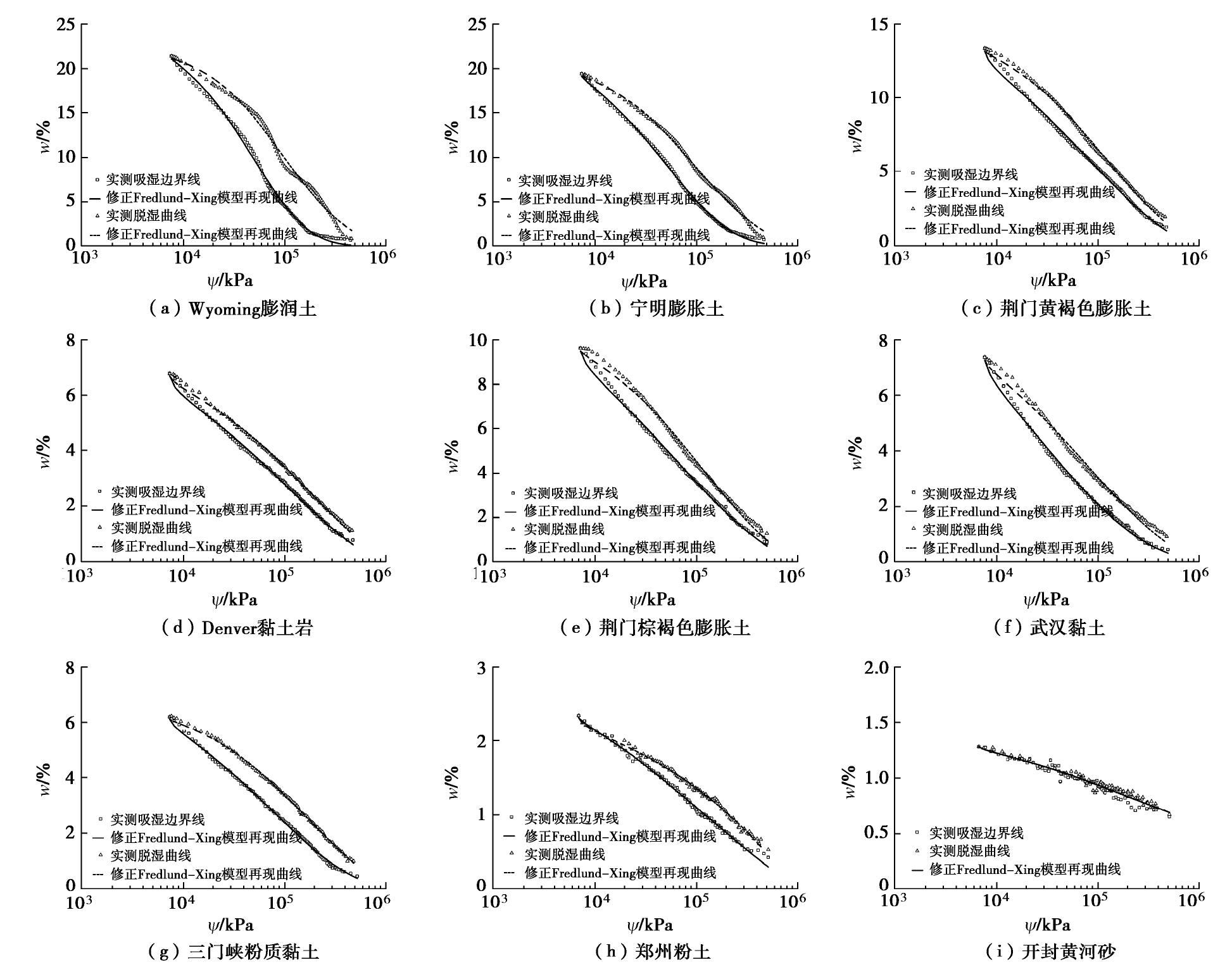
由于Kelvin方程（式(1)）确定了吸力ψ与RH间关系,VSA试验结果既可用w-RH关系亦可用w-ψ关系表达；鉴于非饱和土力学中常用w-ψ关系,因此将9种土样w-ψ关系表达的SWRC绘制在图2中,将每种土样试验历时,试验数据点数,吸湿起始点、吸湿结束点（脱湿起始点）、脱湿结束点对应的RH,ψ,w值列在表2中。

图 2 高吸力下的SWRC（RH = 3%~95%）

Figure 2. Soil-water retention curves under high suctions

下载: 全尺寸图片幻灯片

表 2 高吸力下SWRC的吸湿/脱湿起始点与结束点

Table 2. Start point/end point of adsorption/desorption of SWRCs under high suctions

土样名称	试验历时/min	试验数据点数	吸湿起始点			吸湿结束点（脱湿起始点）			脱湿结束点
土样名称	试验历时/min	试验数据点数	RH	ψ/kPa	w/%	RH	ψ/kPa	w₉₅/%	RH	ψ/kPa	w/%
Wyoming膨润土	3135	203	0.0375	451647	0.67	0.9457	7686	21.46	0.0350	461106	0.87
宁明膨胀土	3777	205	0.0324	471755	0.76	0.9472	7468	19.45	0.0343	463909	1.02
荆门黄褐色膨胀土	2982	204	0.0297	484119	1.28	0.9456	7700	13.40	0.0331	468823	1.96
Denver黏土岩	2113	201	0.0313	476506	0.79	0.9476	7410	6.79	0.0338	465943	1.14
荆门棕褐色膨胀土	2523	212	0.0299	483240	0.89	0.9474	7439	9.63	0.0245	510367	1.28
武汉黏土	2343	202	0.0294	485114	0.44	0.9455	7715	7.37	0.0326	470929	0.91
三门峡粉质黏土	2186	203	0.0244	510892	0.43	0.9478	7380	6.19	0.0327	470498	0.97
郑州粉土	1787	197	0.0246	509791	0.42	0.9500	7061	2.34	0.0245	510347	0.52
开封黄河砂	1295	148	0.0245	510383	0.65	0.9519	6786	1.28	0.0247	509237	0.67

下载: 导出CSV

| 显示表格

$ψ = - \frac{R T}{ν_{w}} \ln RH$ ,

(1)

式中 ψ为吸力（kPa）；R = 8.314 J/(K·mol),为通用气体常数；T为热力学温度,样品室温度25℃对应T = 298.15 K； $ν_{w}$ = 1.8×10^-5 m³/mol,为水的摩尔体积；RH为相对湿度,量纲为1。

由表2可见,9种土样中宁明膨胀土测试历时最长,为3777 min,即2.6 d,且有205个数据点。一方面说明试验历时为文献[14]中饱和盐溶液等压湿度控制法测试历时（319 d）的1/122,极大缩短了测试时间；另一方面说明数据点丰富,能够更好描述土样的持水特征。

从以下3方面探讨高吸力下的SWRC：①反映土样亲水性强弱的持水能力特征点w₉₅；②描述水力滞回效应强弱的SWRC滞回区域面积HHA；③反映水力滞回导致的含水率偏差范围的SWRC滞回效应最大值Δw_max。

2.1 高吸力下持水能力特征点w₉₅

由图2与表2可见：9种土样从完全干燥状态吸湿结束时（控制RH为95%）对应的质量含水率w₉₅差别相当大,高至Wyoming膨润土的21.46%,宁明膨胀土的19.45%,表明持水能力/亲水性非常强,低至开封黄河砂的1.28%,表明其持水能力/亲水性非常弱。由此可见,w₉₅作为RH = 95%下的吸湿质量含水率,是表征土样持水（吸水）能力的特征点,其数值反映了土样亲水性强弱。

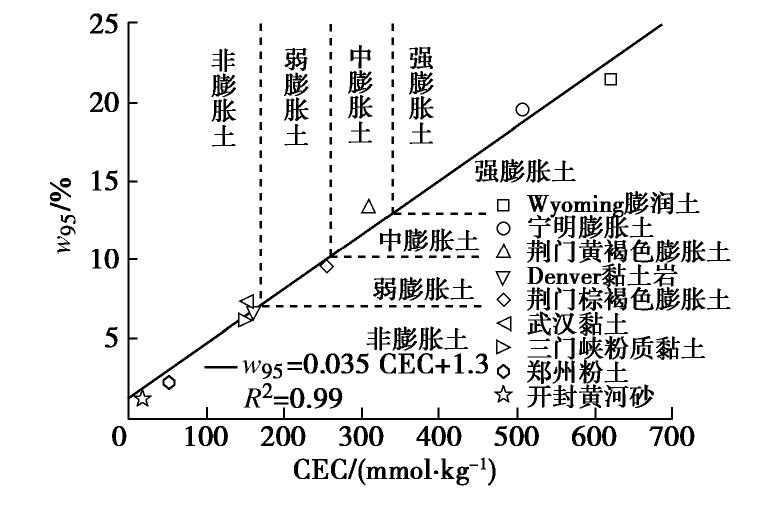
将9种土样的w₉₅与表1给出的CEC对应绘制在图3中,可见w₉₅与CEC具有非常高的线性相关性,其线性拟合结果见式（2）,决定系数R²高达0.99。

$w_{95} = 0.035 CEC + 1.3$ 。

(2)

图 3 9种土的w₉₅-CEC关系

Figure 3. Relationship between w₉₅ and CEC of 9 kinds of soils

下载: 全尺寸图片幻灯片

鉴于《膨胀土地区建筑技术规范》^[21]采用CEC分别为340,260,170 mmol/kg作为判断土样膨胀潜势强（CEC > 340 mmol/kg）、中（CEC =260～340 mmol/kg）、弱（CEC = 170～260 mmol/kg）的界限,将其代入式（2）得到采用w₉₅判别土样膨胀潜势的相应界限分别为13.0%,10.2%,7.1%。即：w₉₅ > 13.0%,判别为强膨胀土；w₉₅=10.2%～13.0%,判别为中膨胀土；w₉₅=7.1%～10.2%,判别为弱膨胀土；w₉₅<7.1%,判别为非膨胀土。

下面对上述方法的可行性进行验证：表3为根据《膨胀土地区建筑技术规范》^[21]测定的Denver膨润土、信阳黏土、商用高岭土的CEC、自由膨胀率及确定的膨胀潜势。采用VSA获得的w₉₅亦列在表3中,Denver膨润土w₉₅为22.47%,大于13.0%,根据w₉₅判别为强膨胀土；信阳黏土w₉₅为8.77%,在7.1%～10.2%,根据w₉₅判别为弱膨胀土；商用高岭土w₉₅为1.03%,小于7.1 %,根据w₉₅判别为非膨胀土。表明采用w₉₅作为膨胀潜势判别标准与采用CEC的判别结果具有很好的一致性,至于如何提高其判别精度,则应收集更多的试验结果,确定更为可靠的强、中、弱膨胀潜势的w₉₅界限值。

表 3 3种土样膨胀潜势的判别指标

Table 3. Eestimation indexes of swelling potential of 3 kinds of soils

土样名称	CEC(NH₄⁺)^[17]/(mmol·kg^-1)	自由膨胀率^[21]/%	膨胀潜势^[21]	w₉₅/%
Denver膨润土	976	111	强	22.47
信阳黏土	206	40	弱	8.77
商用高岭土	4	—	—	1.03

下载: 导出CSV

| 显示表格

2.2 高吸力下SWRC滞回区域面积HHA

由图2可见,高吸力段(RH = 3%～95%),9种土样SWRC均呈现或强或弱的水力滞回行为,即相同吸力下脱湿路径上的w高于吸湿路径上的w。

SWRC水力滞回效应强弱可用脱湿与吸湿曲线间滞回区域面积HHA (hydraulic hysteresis area)描述。根据图2中的脱湿与吸湿曲线数据在Origin软件中数值积分获得9种土样滞回区域面积见表4。

表 4 高吸力下SWRC滞回效应特征参数（R_H = 3%~95%）

Table 4. Hysteresis properties of SWRCs under high suctions

土样名称	滞回区域面积HHA/kPa	HHA 相对值	Δw_max/%	ψ(Δw_max)/kPa	RH(Δw_max)	w_d(Δw_max)/%	w_w(Δw_max)/%	w相对误差/%
土样名称	滞回区域面积HHA/kPa	HHA 相对值	Δw_max/%	ψ(Δw_max)/kPa	RH(Δw_max)	w_d(Δw_max)/%	w_w(Δw_max)/%	$\frac{Δ w_{\max}}{w_{d}}$	$\frac{Δ w_{\max}}{w_{w}}$
Wyoming膨润土	13641	1.00	6.27	68737	0.6069	13.08	6.81	47.9	92.1
宁明膨胀土	10717	0.79	4.18	72744	0.5895	11.00	6.81	38.0	61.4
荆门黄褐色膨胀土	4512	0.33	1.77	37559	0.7612	9.87	8.10	17.9	21.9
Denver黏土岩	2367	0.17	0.69	37184	0.7633	4.80	4.11	14.4	16.8
荆门棕褐色膨胀土	3520	0.26	1.38	30993	0.7984	7.41	6.03	18.6	22.9
武汉黏土	3412	0.25	1.15	31077	0.7979	5.13	3.98	22.4	28.9
三门峡粉质黏土	3439	0.25	1.04	89267	0.5228	3.55	2.51	29.3	41.4
郑州粉土	1002	0.07	0.37	155335	0.3235	1.22	0.85	30.3	43.5
开封黄河砂	196	0.01	0.16	221700	0.1999	0.88	0.72	18.2	22.2

下载: 导出CSV

| 显示表格

由图2与表4可见：9种土样HHA差别显著,对于强膨胀土,Wyoming膨润土高达13641 kPa,宁明膨胀土亦高达10717 kPa；对于中膨胀土,荆门黄褐色膨胀土为4512 kPa；对于弱膨胀土,荆门棕褐色膨胀土为3520 kPa；武汉黏土、三门峡粉质黏土亦达到3412,3439 kPa；而郑州粉土低至1002 kPa；开封黄河砂仅有196 kPa,是Wyoming膨润土的1/70,滞回效应可忽略不计。

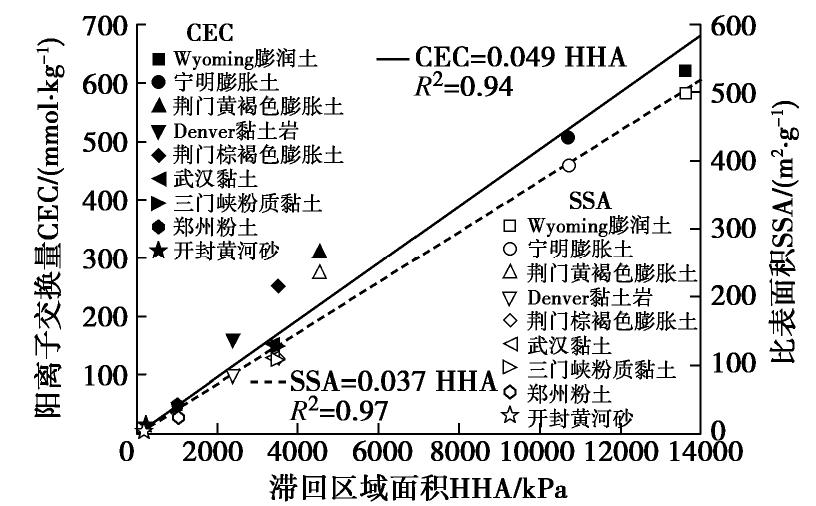
以上数据表明：HHA与土样膨胀潜势正相关,将9种土样的HHA与表1给出的CEC、SSA对应绘制在双y轴坐标系中,见图4,可见HHA与CEC、SSA均具有非常高的线性相关性,其过坐标原点的线性拟合结果为

图 4 9种土的CEC-SSA-HHA关系

Figure 4. CEC-SSA-HHA relationship of 9 kinds of soils

下载: 全尺寸图片幻灯片

$CEC = 0 .049 HHA$ ,

(3)

$SSA = 0 .037 HHA$ ,

(4)

式中,HHA与CEC线性相关的R²达到0.94,HHA与SSA线性相关的R²高达0.97。

CEC与SSA是强烈相关但有明显区别的土性指标。CEC指单位质量土样吸附的可交换阳离子总量,反映由于颗粒带负电荷导致的土样吸附阳离子的能力,更大的CEC意味着更强的膨胀潜势。SSA指单位质量土样具有的表面积,反映土样的颗粒级配与矿物成分,更大的SSA意味着更大的塑性,更强的膨胀潜势,更强的亲水性。HHA与CEC、SSA均正相关表明,滞回效应大小既受土样吸附阳离子能力的强烈影响,亦受土样比表面积控制,且从R²上看,其受土样比表面积影响更大。

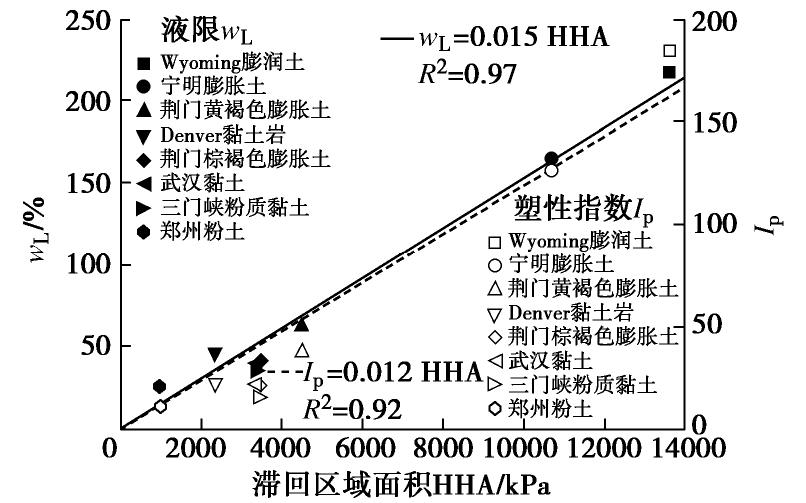
将8种细粒土HHA与其液限w_L、塑性指数I_p绘制在双y轴坐标系中,见图5。

图 5 8种细粒土的w_L-I_p-HHA关系

Figure 5. w_L-I_p-HHA relationship of 8 kinds of fine-grained soils

下载: 全尺寸图片幻灯片

可见HHA与w_L、I_p均正相关,过坐标原点的线性拟合结果分别为

$w_{L} = 0 .015 HHA$ ,

(5)

$I_{p} = 0.012 HHA$ 。

(6)

式中,HHA与w_L线性相关的R²高达0.97,HHA与I_p线性相关的R²亦达到0.92,表明细粒土HHA与w_L具有更高的线性相关性。由于w_L、I_p代表土样塑性大小,表明HHA受土样塑性大小控制。

2.3 高吸力下SWRC滞回效应最大值Δw_max

SWRC滞回效应指水力滞回（脱/吸湿路径）对SWRC（此处是w-ψ关系）的影响,此处SWRC滞回效应最大值定义为相同ψ下,脱湿曲线对应含水率w_d与吸湿曲线对应含水率w_w之差的最大值Δw_max。根据该定义,在图2中搜索到每种土的Δw_max,及其对应的吸力ψ、RH、脱湿曲线含水率w_d、吸湿曲线含水率w_w,列入表4中。

由表4与图2可见,9种土样Δw_max存在较大差异：强膨胀土Δw_max相当大,如Wyoming膨润土为6.27%,宁明膨胀土为4.18%；中膨胀土,荆门黄褐色膨胀土为1.77 %；弱膨胀土,荆门棕褐色膨胀土为1.38%；中等塑性黏土,武汉黏土为1.15%,三门峡粉质黏土为1.04%,Denver黏土岩为0.69%；低塑性黏土,郑州粉土为0.37%；开封黄河砂仅为0.16%。对比表1可见,土样Δw_max值与w_L,I_p,CEC,SSA均正相关,反映了水力滞回导致的含水率偏差范围。取Δw_max与相应w_d、w_w的比值作为SWRC的含水率相对误差,计算9种土样Δw_max/w_d、Δw_max/w_w结果列于表4与图6。

图 6 由于水力滞回导致的含水率相对误差

Figure 6. Relative errors of water content due to hydraulic hysteresis

下载: 全尺寸图片幻灯片

表4与图6中,同一土样w_w小于w_d,因此采用吸湿曲线含水率w_w作为分母的相对误差比采用脱湿曲线含水率w_d作为分母的相对误差要大。由相对误差计算结果可见：在高吸力范围,不考虑水力滞回会导致持水特征曲线SWRC上质量含水率w最高92.1 %（Wyoming膨润土）的相对误差。

另一方面,如果工程中需要用当前含水率估算吸力,则同一w下,脱湿路径上吸力与吸湿路径上吸力的比值最高可达2.71（图2中Wyoming膨润土,w为6%,脱湿路径上吸力205959 kPa,吸湿路径上吸力76119 kPa,比值为2.71）,印证了高吸力下强膨胀土SWRC滞回效应之强烈。

2.4 高吸力下SWRC滞回行为机制探讨

由图2及2.2节、2.3节对SWRC滞回区域面积HHA与滞回效应最大值Δw_max的分析,可知高吸力下,9种土样中,高塑性（液限高、塑性指数大）、阳离子交换量大、比表面积大的土样滞回效应大,在此对高吸力下SWRC滞回行为机制展开探讨。

水力滞回行为机制解释主要有墨水瓶效应^[1]与接触角效应^[13],前者由于土中孔隙尺寸与形状（可用孔径r概化）的不均匀性所致,后者由于吸湿接触角 $α_{w}$ 通常大于脱湿接触角 $α_{d}$ 所致^[23]。墨水瓶效应与接触角效应均属毛细范畴,受Young-Laplace方程控制：

$ψ_{c} = u_{a} - u_{w} = \frac{2 T_{s} \cos α}{r}$ 。

(7)

式中 ψ_c为毛细吸力,可用孔隙气压力u_a与孔隙水压力u_w之差表达；T_s为水的表面张力； $α$ 为接触角；r为毛细半径。当吸力增大、土中水能量状态低到其空化点以下,毛细水汽化,（土中毛细水比自由水空化点更低,但其对应的ψ_c或RH尚无定论,一般而言RH低于80%,毛细水难以存在^[2]）,墨水瓶效应与接触角效应难以解释高吸力下的滞回行为。

Lu等^[24]给出了高吸力下SWRC滞回机制的一种解释：非膨胀黏土,脱湿与吸湿主要发生在颗粒表面,因而滞回效应较小。膨胀性土,脱湿与吸湿亦可发生于存在可交换阳离子的晶层之间,吸湿时阳离子水化吸附极性水分子,水分子需克服较大的,van der Waals力方能进入层间；脱湿时由于层间距离较大van der Waals力较弱,因此相同ψ下,吸湿路径对应较小的w,脱湿路径对应较大的w。在此结合文中数据探讨该解释的合理性：

高吸力下Wyoming膨润土HHA=13641 kPa,Δw_max=6.27 %,滞回效应最大；自由膨胀率为775 %,膨胀性最大；SSA高达500.1 m²/g,表明其包含了较大的层间比表面积^[25],CEC高达621 mmol/kg,表明其可交换阳离子总量大；印证了吸湿状况下膨胀土层间阳离子水化受van der Waals力阻碍致其含水率较低的机制解释。

相对而言,郑州粉土,SSA为27.7 m²/g,CEC为50 mmol/kg,比表面积小,可交换阳离子总量小。一方面其自由膨胀率（9%）为Wyoming膨润土的1.2 %,表明其为非膨胀土；另一方面,其HHA（1002 kPa）为Wyoming膨润土的7%,Δw_max（0.37%）为Wyoming膨润土的6%,滞回效应很小。印证了非膨胀土脱湿吸湿主要发生在颗粒表面（而非层间）致其滞回效应较小的机制解释。

以上讨论明晰了2.2节中的论述：高吸力下滞回效应既受CEC的强烈影响,亦受SSA控制,且从R²上看,其受SSA影响更大。至于是否考虑滞回效应,应视土样与工况等具体情况而定,鉴于Wyoming膨润土具有最大的HHA,可采用其作为标准,其它土样的HHA相对值亦列入表4中,可见从开封黄河砂的0.01到宁明膨胀土的0.79,HHA相对值可作为实际工况中是否考虑滞回效应的依据。

3. 高吸力下持水特征的本构描述

基于对以上9种土样高吸力下SWRC规律性的认知,经尝试,建议了Fredlund-Xing（1994）模型^[1]的修正形式描述高吸力下SWRC：

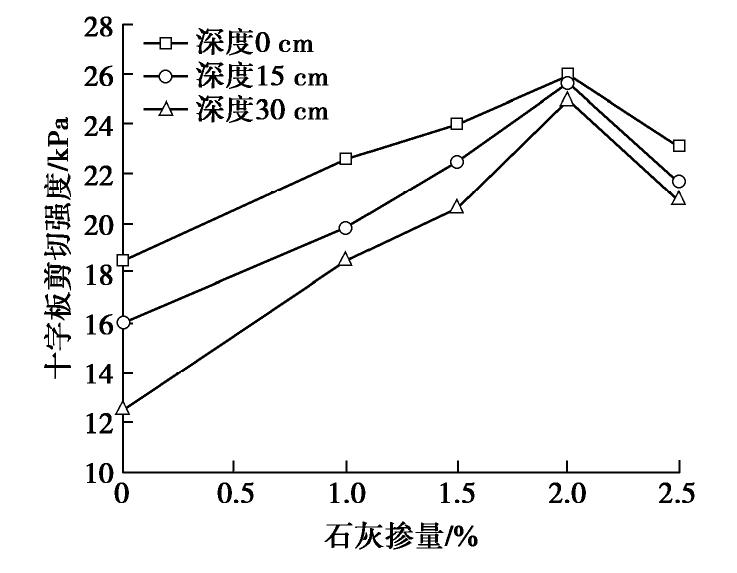
$\begin{matrix} w (ψ) = C (ψ) \frac{w_{u}}{{\ln [\exp (1) + {(\frac{(ψ - ψ_{b})}{a})}^{n}]}^{m}} \\ = [1 - \frac{\ln (1 + ψ / ψ_{r})}{\ln (1 + 10^{6} / ψ_{r})}] \frac{w_{u}}{{\ln [\exp (1) + {(\frac{(ψ - ψ_{b})}{a})}^{n}]}^{m}} 。 \end{matrix}$

(8)

式中 w为质量含水率（%）；ψ为吸力（kPa）；w(ψ)为质量含水率表达的SWRC；C(ψ)为修正系数函数,能够迫使SWRC通过零含水率对应吸力10⁶ kPa的点。4个参数a,n,m,ψ_r为Fredlund-Xing(1994)模型已有参数,a为与拐点位置相关的土性参数（kPa）；n为与拐点处斜率相关的土性参数,量纲为1,m为与曲率相关的土性参数,量纲为1,ψ_r为残余吸力（kPa）。

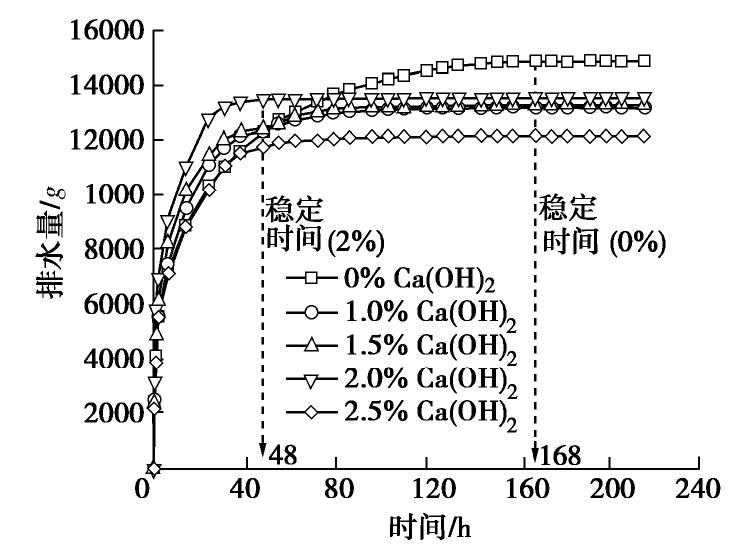
为描述高吸力下SWRC,将Fredlund-Xing模型中饱和质量含水率w₀用w_u替代,w_u为高吸力下SWRC质量含水率上边界（最大值）,相应吸力为ψ_b。增加土性参数ψ_b是式（8）与Fredlund-Xing模型形式上的主要区别,ψ_b单位为kPa,物理意义是高吸力下SWRC吸力下边界。由此可见,修正的Fredlund-Xing模型共有6个参数：w_u,ψ_b,ψ_r,a,n,m。为区分吸湿与脱湿过程,相应参数增加上标w（wetting,表示吸湿）或d（drying,表示脱湿）。

采用式（8）分别对9种土样高吸力下SWRC进行拟合,流程如下：

（1）采用表2给出的吸湿结束点（脱湿起始点）含水率w₉₅值作为w_u值,对应吸力值作为ψ_b值；w_u与ψ_b为吸脱湿过程共用参数值。

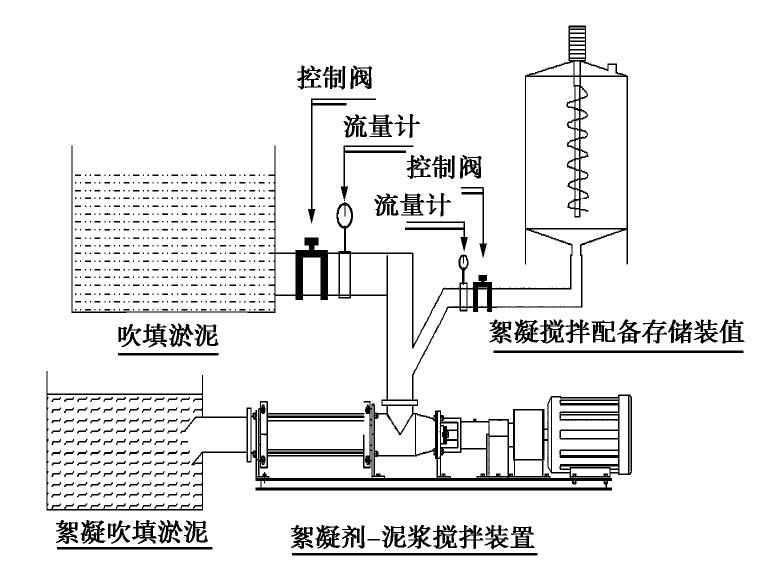
（2）对于吸湿过程,采用表2给出的吸湿起始点吸力值作为ψ_r值；至此,吸湿过程所需确定的参数仅余a,n,m。依据式（8）在Excel中通过规划求解功能对吸湿过程SWRC数据进行非线性拟合获得a,n,m最优解以及拟合结果的决定系数R²。

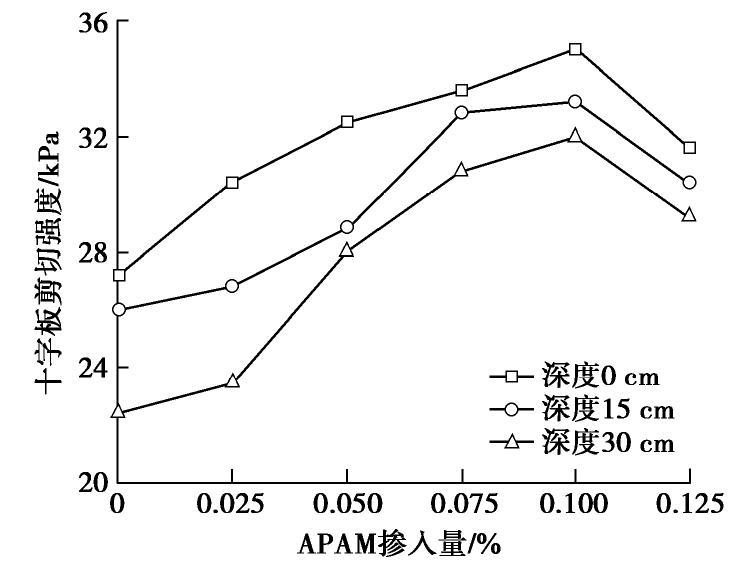
（3）对于脱湿过程,采用表2给出的脱湿结束点吸力值作为ψ_r值；依据式（8）在Excel中通过规划求解功能对脱湿过程SWRC数据进行非线性拟合获得a,n,m最优解以及R²。

前8种土样采用上述流程获得的模型参数与R²见表5,可见8种土样,无论脱湿吸湿,修正的Fredlund-Xing模型再现结果的R²均在0.99以上。为节约篇幅,其修正Fredlund-Xing模型数值再现结果亦放在图2中,可见式（8）相当好地再现了高吸力下8种土样脱湿与吸湿过程SWRC。

表 5 高吸力下修正Fredlund-Xing模型参数

Table 5. Modified Fredlund-Xing model parameters for 9 kinds of soils under high suctions

土样名称	共用参数		吸湿过程					脱湿过程
土样名称	w_u/%	ψ_b/kPa	ψ_r^w/kPa	a^w/kPa	n^w	m^w	R²	ψ_r^d/kPa	a^w/kPa	n^d	m^d	R²
Wyoming膨润土	21.46	7686	451647	248967	0.94	10.54	1.00	461106	90593	1.06	2.09	0.99
宁明膨胀土	19.45	7468	471755	245967	0.82	8.02	1.00	463909	90230	0.94	2.13	1.00
荆门黄褐色膨胀土	13.40	7700	484119	237692	0.61	4.34	1.00	468823	91452	0.82	1.90	1.00
Denver黏土岩	6.79	7410	476506	267853	0.57	4.14	1.00	465943	91075	0.66	1.79	1.00
荆门棕褐色膨胀土	9.63	7439	483240	87263	0.64	2.81	1.00	510367	86362	0.80	1.96	0.99
武汉黏土	7.37	7715	485114	81572	0.67	3.77	1.00	470929	80590	0.71	2.39	0.99
三门峡粉质黏土	6.19	7380	510892	213682	0.67	4.50	1.00	470498	91368	0.82	1.56	1.00
郑州粉土	2.34	7061	509791	30152	0.78	1.17	0.99	510347	31576	0.58	0.86	1.00
开封黄河砂	1.28	6786	—	76542	0.66	1.04	0.96	—	76542	0.66	1.04	0.96

下载: 导出CSV

| 显示表格

对第9种土样开封黄河砂,由图2可见其SWRC滞回行为非常小,因此采用同一组模型参数描述其SWRC,此外不考虑式（8）中修正系数函数C(ψ),即C(ψ) = 1,对吸脱湿SWRC数据进行非线性拟合获得a,n,m最优解见表5,同时描述其吸脱湿SWRC的R²达到0.96,由图2的再现结果可见式（8）非常好地对其SWRC进行了本构描述。

此外,对比表5中吸脱湿过程a,n,m值可见,对同种土样,相对脱湿过程,吸湿过程a值较大说明其拐点位置靠后,n值较低说明其拐点处斜率相对较小,m值较大说明其曲率较大。

由此可见,文中建议的Fredlund-Xing模型修正形式有6个参数：w_u,ψ_b,ψ_r值直接从实测SWRC数据确定,a,n,m值通过非线性拟合获得。Fredlund- Xing(1994)模型中4个参数（a,n,m,ψ_r）需通过非线性拟合获得,还有一个参数饱和质量含水率w₀需试验测定（从实测SWRC数据确定）,实际上有5个参数。文中建议的修正形式仅增加了一个参数ψ_b,且ψ_b与w_u、ψ_r直接从实测SWRC数据确定,只需非线性拟合3个参数（a,n,m）,且该修正形式能够很好地分别描述吸湿与脱湿过程中SWRC强烈的非线性行为。

4. 结论

（1）9种土样高吸力下吸湿与脱湿过程持水特征测试最长历时为2.6 d,有205个数据点,说明动态露点法极大缩短了高吸力下SWRC测试时间,且数据点丰富,能够更好描述土样持水特征。

（2）w₉₅作为RH = 95%下的吸湿质量含水率,是表征土样持水能力的特征点,反映土样亲水性强弱,w₉₅与CEC具有非常高的线性相关性,w₉₅作为膨胀潜势的判别标准是可行的。

（3）SWRC水力滞回效应强弱可用吸脱湿曲线间滞回区域面积HHA描述,HHA与CEC、SSA均有非常高的线性相关性,表明滞回效应强弱既受土样吸附阳离子能力的强烈影响,亦受土样SSA控制,且从R²看,其受SSA影响更大。HHA与w_L,I_p均正相关,表明HHA受土样塑性大小控制。

（4）SWRC滞回效应最大值可用同一ψ下脱湿与吸湿曲线对应w之差最大值Δw_max量化,土样Δw_max值与w_L,I_p,CEC,SSA均正相关,反映了水力滞回导致的w偏差范围。高吸力范围,不考虑水力滞回会导致SWRC上w最高92.1 %的相对误差。如工程中需要用当前w估算ψ,则同一w下,脱湿路径上ψ与吸湿路径上ψ的比值最高可达2.71。

（5）建议采用Fredlund-Xing模型的修正形式描述高吸力下SWRC,方程有6个参数,w_u,ψ_b,ψ_r值直接从实测SWRC数据确定,a,n,m值通过非线性拟合获得。对9种土样SWRC的修正Fredlund -Xing模型数值再现结果表明该方程可分别很好地描述吸湿与脱湿过程中SWRC强烈的非线性行为。

致谢: 感谢土力学和岩土工程界各位同行的信任,让我有幸成为今年黄文熙讲座的主讲人；本文研究和撰写过程中得到了浙江工业大学土木工程学院孙宏磊教授、曾玲玲教授、潘晓东副教授、史吏副教授、徐山琳博士后,温州大学建筑工程学院王军教授、王鹏教授、符洪涛副教授等的大力帮助,也部分反映了课题组的研究成果。笔者的相关研究生论文成果是本文的主要基础,这些研究生包括翁振奇、刘斯杰、何自立、陆靖凌、陆逸、张皓、朱彦臻。感谢国家自然科学基金委对笔者相关研究的持续支持。

图 1 不同初始含水率温州淤泥的e- $\lg {σ^{'}}_{v}$ 压缩曲线

Figure 1. e- $\lg {σ^{'}}_{v}$ curves of Wenzhou sludge with different initial water contents

下载: 全尺寸图片幻灯片

图 2 不同淤泥在相近初始含水率下的e- $\lg {σ^{'}}_{v}$ 压缩曲线

Figure 2. e- $\lg {σ^{'}}_{v}$ curves of different sludges with similar initial water content

下载: 全尺寸图片幻灯片

图 3 重塑屈服压力与不同淤泥 $e_{0} / e_{L}$ 归一化关系

Figure 3. Normalized relationship between yield stress and $e_{0} / e_{L}$

下载: 全尺寸图片幻灯片

图 4 不同初始含水率吹填淤泥的k_v- $\lg {σ^{'}}_{v}$ 渗透曲线

Figure 4. k_v- $\lg {σ^{'}}_{v}$ curves of sludge with different initial water contents

下载: 全尺寸图片幻灯片

图 5 不同初始含水率温州淤泥的k_v- $\lg {σ^{'}}_{v}$ 曲线^[16]

Figure 5. k_v- $\lg {σ^{'}}_{v}$ curves of Wenzhou sludge with different initial water contents^[16]

下载: 全尺寸图片幻灯片

图 6 k_v- $\lg {σ^{'}}_{v}$ 曲线试验结果与计算结果的对比^[16]

Figure 6. Comparison between calculated and test results of k_v- $\lg {σ^{'}}_{v}$ ^[16]

下载: 全尺寸图片幻灯片

图 7 相近液限淤泥的k_v-e曲线^[16]

Figure 7. k_v-e curves of sludge with similar liquid limit ^[16]

下载: 全尺寸图片幻灯片

图 8 不同液限淤泥的双对数坐标下的k_v-e关系^[16]

Figure 8. k_v- e relationship of sludge with different liquid limits under log-log coordinate^[16]

下载: 全尺寸图片幻灯片

图 9 试验仪器的示意图

Figure 9. Schematic arrangement of test apparatus

下载: 全尺寸图片幻灯片

图 10 吹填淤泥位移场

Figure 10. Displacement fields of sludge

下载: 全尺寸图片幻灯片

图 11 第142小时的应变路径

Figure 11. Strain paths at 142nd h

下载: 全尺寸图片幻灯片

图 12 应变路径与时间的关系

Figure 12. Relationship between strain paths and time

下载: 全尺寸图片幻灯片

图 13 ε_p-ε_q坐标中的应变路径(其中ε_p=ε_v+ε_r; ε_q=(ε_v-ε_r)/2)

Figure 13. Strain paths in ε_p-ε_q coordinates (ε_p=ε_v+ε_r; ε_q=(ε_v-ε_r)/2)

下载: 全尺寸图片幻灯片

图 14 观察窗位置分布

Figure 14. Configuration of observation windows

下载: 全尺寸图片幻灯片

图 15 0~3 h内的位移场变化云图

Figure 15. Contours of displacement field within 0~3 h

下载: 全尺寸图片幻灯片

图 16 3~142 h内的位移场变化云图

Figure 16. Contours of displacement field within 3~142 h

下载: 全尺寸图片幻灯片

图 17 土柱半径随时间变化曲线

Figure 17. Variation of radius of soil columns with time

下载: 全尺寸图片幻灯片

图 18 颗粒的无量纲位移

Figure 18. Dimensionless displacements of particles

下载: 全尺寸图片幻灯片

图 19 颗粒的无量纲径向位移

Figure 19. Dimensionless radial displacements of particles

下载: 全尺寸图片幻灯片

图 20 土柱半径随时间变化

Figure 20. Variation of radius of soil columns with time

下载: 全尺寸图片幻灯片

图 21 孔隙水压力消散曲线

Figure 21. Curves of pore water pressure dissipation

下载: 全尺寸图片幻灯片

图 22 不同种类淤泥的粒径分布

Figure 22. Particle-size distributions of different sludges

下载: 全尺寸图片幻灯片

图 23 不同种类淤泥的土柱半径随时间变化

Figure 23. Variation of radius of soil columns of different sludges with time

下载: 全尺寸图片幻灯片

图 24 不同种类土体的孔隙水压力消散曲线

Figure 24. Curves of pore water pressure dissipation of different sludges

下载: 全尺寸图片幻灯片

图 25 土体在每一个24 h内的位移变化云图

Figure 25. Displacement patterns of sludge in each 24 h

下载: 全尺寸图片幻灯片

图 26 径向、竖向真空度时空变化的大应变固结计算简图

Figure 26. Analysis scheme of unit cell with vertical drains considering variation of vacuum pressure in radial and vertical directions

下载: 全尺寸图片幻灯片

图 27 径竖向真空度时间因子对固结的影响

Figure 27. Consolidation of cases with various k_r2 and k_z2

下载: 全尺寸图片幻灯片

图 28 不同加固深度下径竖向真空度衰减的影响

Figure 28. Consolidation of cases with different soil thicknesses

下载: 全尺寸图片幻灯片

图 29 不同径向真空度衰减下超静孔压径向分布

Figure 29. Radial distribution of excess pore water pressure under different radial losses

下载: 全尺寸图片幻灯片

图 30 不同时间因子对小应变固结与大应变固结的影响

Figure 30. Comparison between small and large strain solutions

下载: 全尺寸图片幻灯片

图 31 考虑土柱的不均匀应变固结计算简图

Figure 31. Analysis scheme of unit cell with vertical drains considering soil columns

下载: 全尺寸图片幻灯片

图 32 排水板通水量衰减速度对两区域固结的影响

Figure 32. Effects of decay speed of discharge capacity on consolidation degree for soil columns and normal zone

下载: 全尺寸图片幻灯片

图 33 土柱区与外围渗透系数比对两区域固结的影响

Figure 33. Effects of permeability ratio on consolidation degree for soil columns and normal zone

下载: 全尺寸图片幻灯片

图 34 土柱区与外围压缩系数比对两区域沉降的影响

Figure 34. Effects of compressibility ratio on settlements for soil columns and normal zone

下载: 全尺寸图片幻灯片

图 35 土柱半径对两区域固结的影响

Figure 35. Effects of radius of soil columns on consolidation degree for soil columns and normal zone

下载: 全尺寸图片幻灯片

图 36 技术路线图

Figure 36. Technological roadmap

下载: 全尺寸图片幻灯片

图 37 排水板

Figure 37. PVD

下载: 全尺寸图片幻灯片

图 38 试验装置图

Figure 38. Diagram of test apparatus

下载: 全尺寸图片幻灯片

图 39 真空度随时间变化曲线

Figure 39. Variation of vacuum pressure

下载: 全尺寸图片幻灯片

图 40 含水率和十字板剪切强度随深度变化曲线

Figure 40. Variation of water content and vane shear strength with depth

下载: 全尺寸图片幻灯片

图 41 试验完成后土中挖出的排水板实拍照片

Figure 41. PVDs after tests

下载: 全尺寸图片幻灯片

图 42 梯度比试验装置示意图

Figure 42. Schematic diagram of GR test apparatus

下载: 全尺寸图片幻灯片

图 43 不同水力梯度下G_R随时间的变化曲线

Figure 43. Curves of G_R with time at different hydraulic gradients

下载: 全尺寸图片幻灯片

图 44 不同孔径滤膜的排水量随时间变化

Figure 44. Variation of volume of drainage with time for different filters

下载: 全尺寸图片幻灯片

图 45 十字板剪切强度随深度和距排水板径向距离变化曲线

Figure 45. Vane shear strengths at different depths and distances from PVD

下载: 全尺寸图片幻灯片

图 46 表面沉降随时间的变化

Figure 46. Variation of surface settlement with time

下载: 全尺寸图片幻灯片

图 47 最大沉降差随时间的变化

Figure 47. Variation of maximum settlement difference with time

下载: 全尺寸图片幻灯片

图 48 增压管样式图

Figure 48. Illustration of booster pipeline

下载: 全尺寸图片幻灯片

图 49 真空和增压压力作用下土单元的应力状态

Figure 49. Stress states of soil element subjected to vacuum pressure and booster pressure

下载: 全尺寸图片幻灯片

图 50 深度50 cm处孔隙水压力变化

Figure 50. Variation of pore water pressure at depth of 50 cm

下载: 全尺寸图片幻灯片

图 51 含水率和十字板剪切强度随深度的变化趋势

Figure 51. Variation of water content and vane shear strength with depth

下载: 全尺寸图片幻灯片

图 52 增压式真空预压法示意图

Figure 52. Schematic layout of air booster vacuum preloading system

下载: 全尺寸图片幻灯片

图 53 现场试验布置图

Figure 53. Layout of in-situ test site

下载: 全尺寸图片幻灯片

图 54 不同深度孔隙水压力的变化

Figure 54. Variationof pore water pressure at different depths

下载: 全尺寸图片幻灯片

图 55 两个区域的地表沉降量随时间变化曲线

Figure 55. Variation of surface settlements in two zones

下载: 全尺寸图片幻灯片

图 56 A区和B区初始和加固后的土体含水率曲线

Figure 56. Initial and post-soil improvement water content profiles for Zone A and Zone B

下载: 全尺寸图片幻灯片

图 57 A区和B区初始和加固后的土体十字板剪切强度曲线

Figure 57. Initial and post-soil improvement vane shear strength profiles for Zone A and Zone B

下载: 全尺寸图片幻灯片

图 58 不同石灰掺量下排水量随时间变化曲线

Figure 58. Variation of discharged water with time under various lime contents

下载: 全尺寸图片幻灯片

图 59 不同石灰含量十字板强度随深度变化曲线

Figure 59. Variation of vane shear strength with depth under various lime contents

下载: 全尺寸图片幻灯片

图 60 不同APAM掺量下十字板剪切强度

Figure 60. Vane shear strengths at different doses of APAM

下载: 全尺寸图片幻灯片

图 61 絮凝搅拌装置

Figure 61. Device of flocculation and mixing

下载: 全尺寸图片幻灯片

图 62 地表沉降量变化曲线

Figure 62. Variation of surface settlement

下载: 全尺寸图片幻灯片

图 63 距PVD径向距离0.05 m处十字板强度随深度变化曲线

Figure 63. Variation of vane shear strength at radial distance of 0.05 m with depth

下载: 全尺寸图片幻灯片

图 64 锥头阻力剖面图

Figure 64. Cone resistance profiles

下载: 全尺寸图片幻灯片

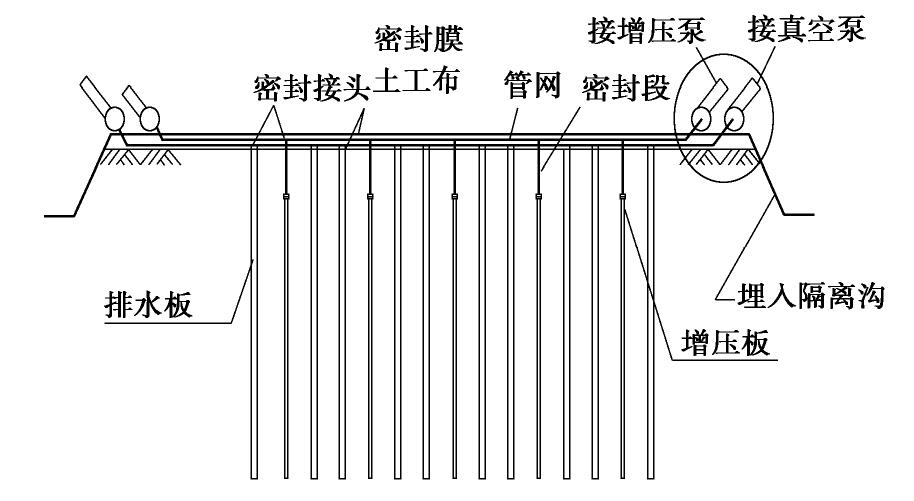
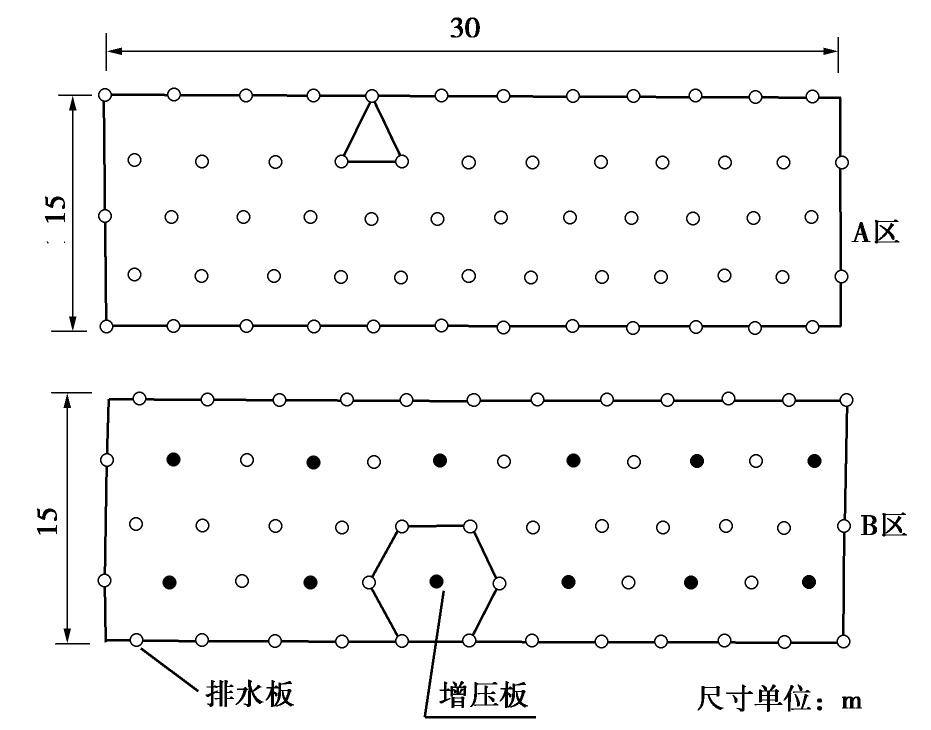
表 1 淤泥的初始含水率

Table 1 Initial water contents of sludges

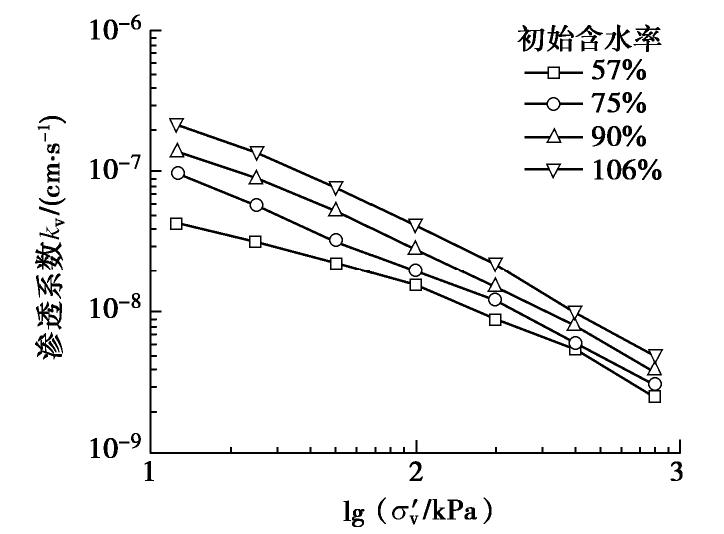
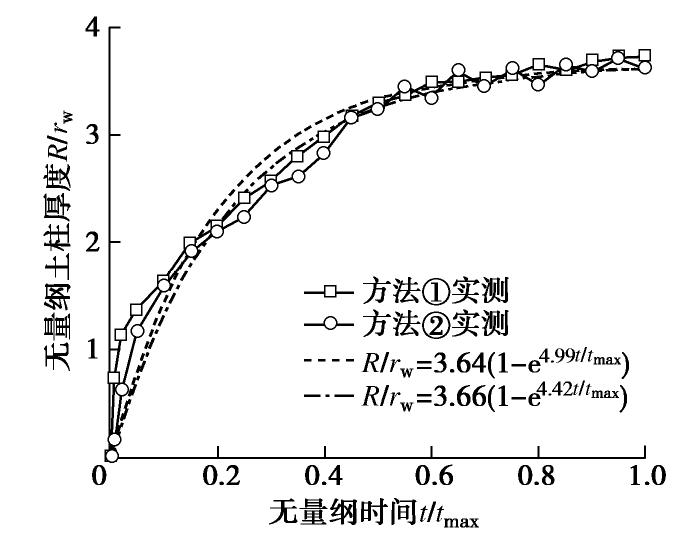
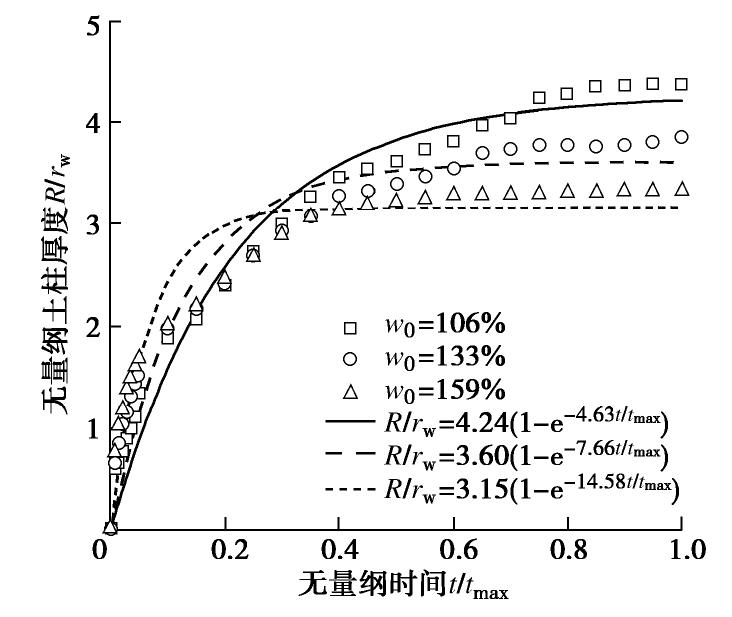
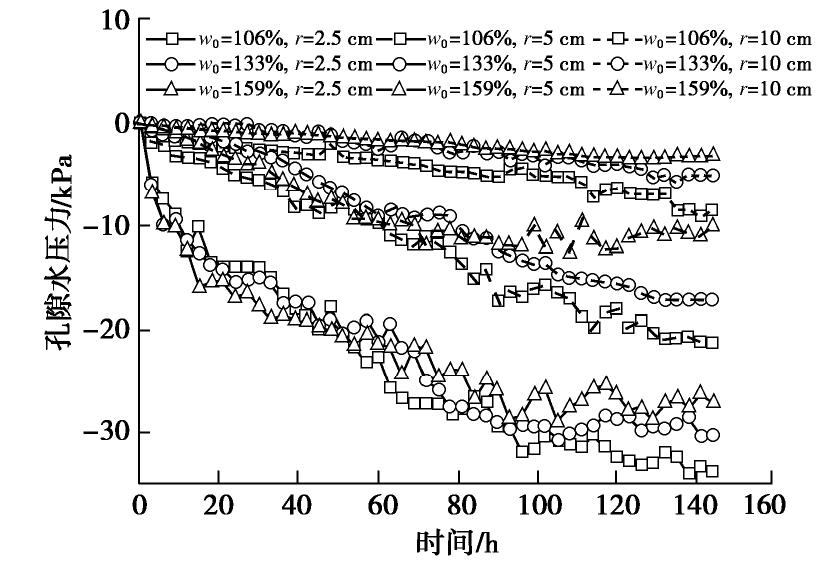
试验编号	$w_{0}$ /%	$w_{L}$ /%	$w_{0}$ / $w_{L}$
1	106	53	2.0
2	133	53	2.5
3	159	53	3.0

下载: 导出CSV

表 2 淤泥的初始含水率

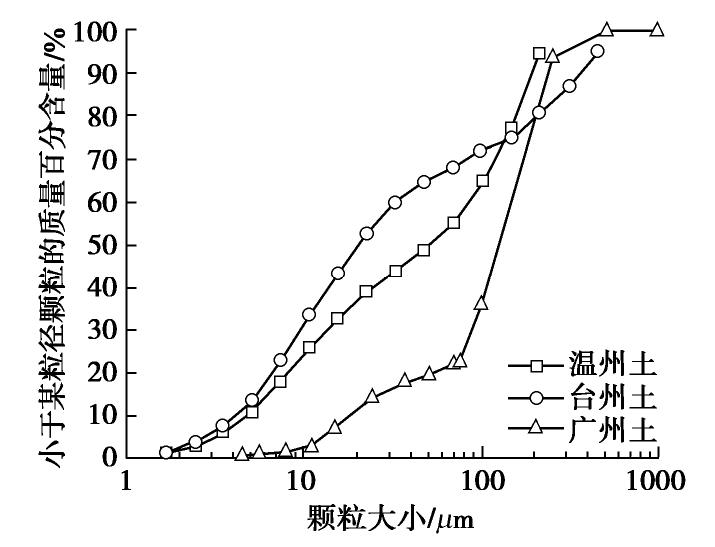
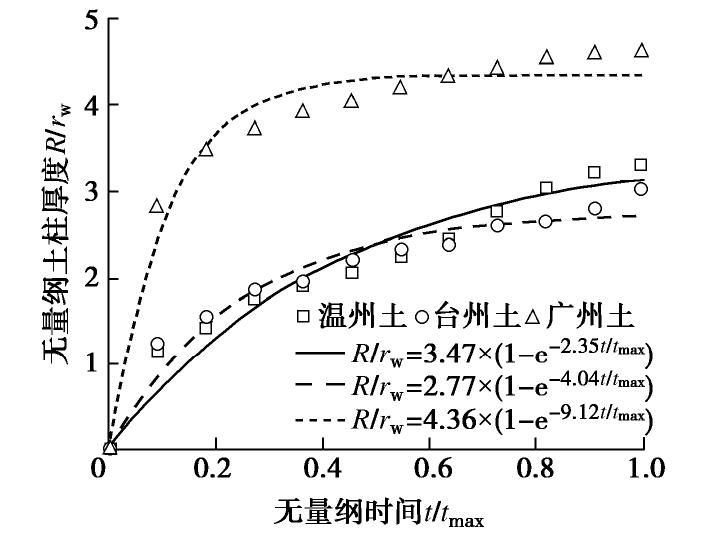
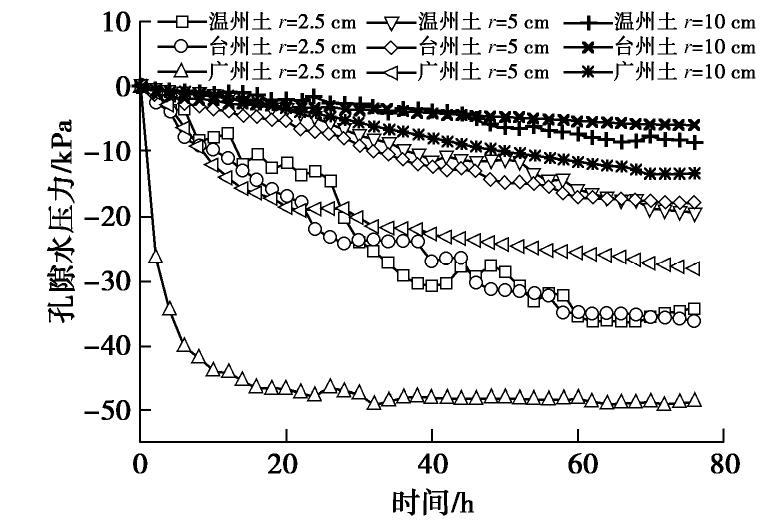
Table 2 Initial water contents of sludges

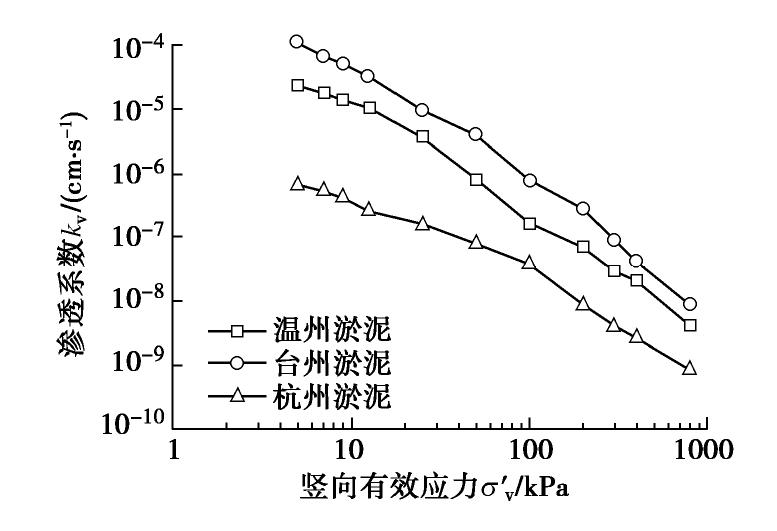
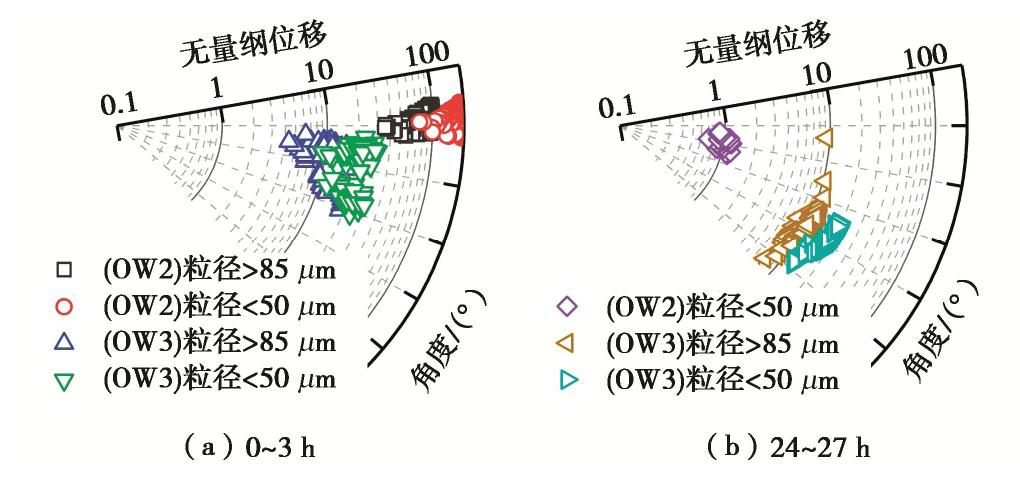
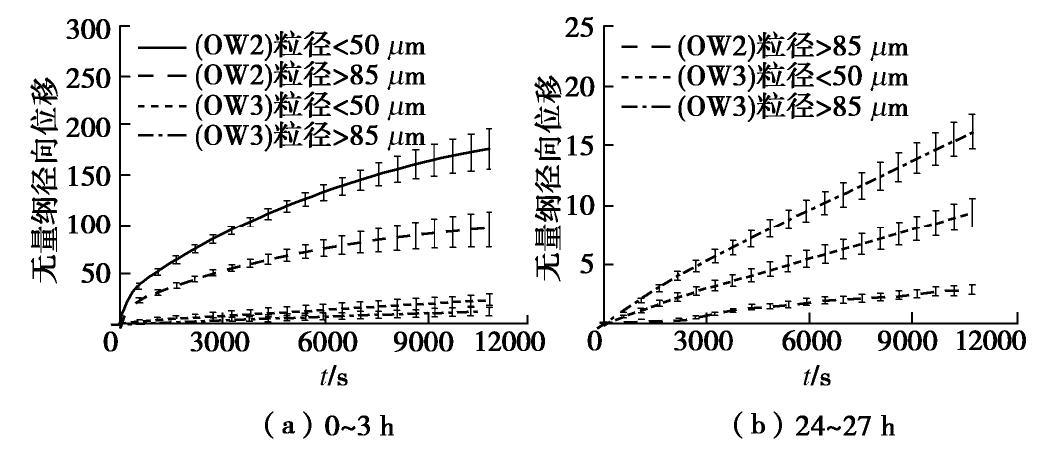
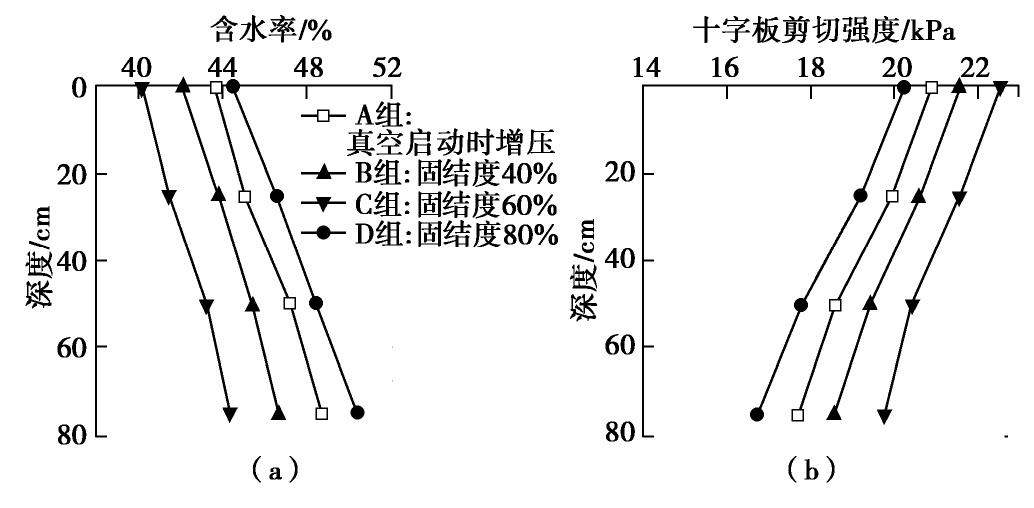
试验土样	$w_{0}$ /%	$w_{L}$ /%	$w_{0}$ / $w_{L}$
温州土	106	53	2
台州土	80	40	2
广州土	54	27	2

下载: 导出CSV

表 3 试验方案

Table 3 Test schemes

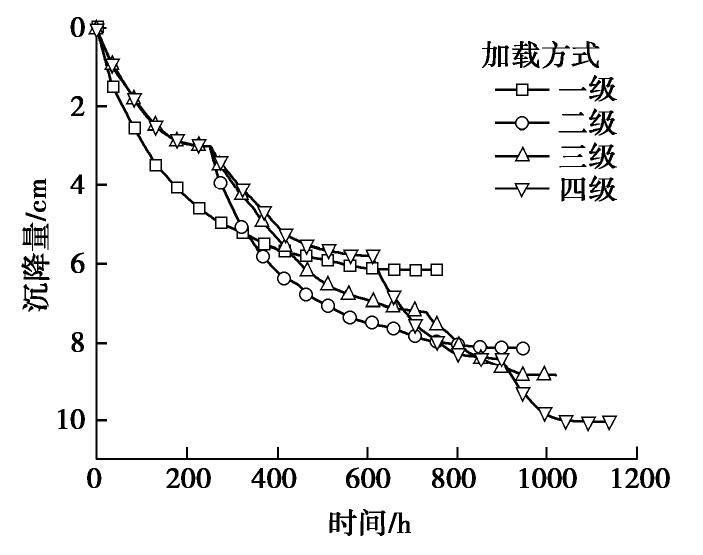
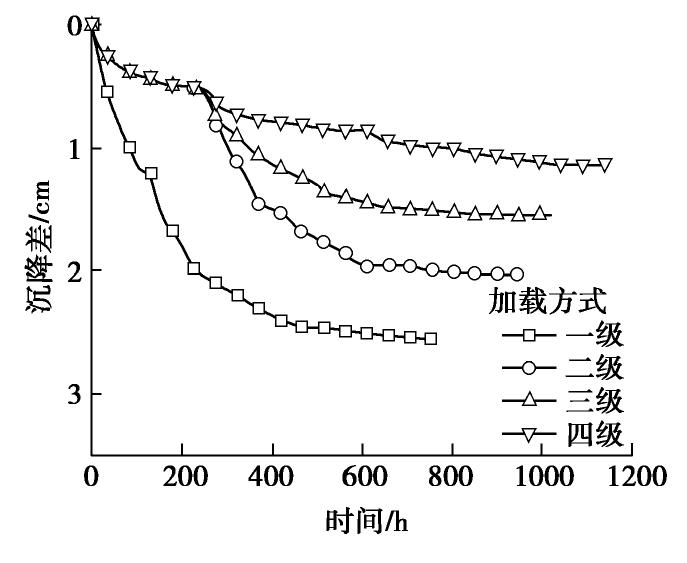
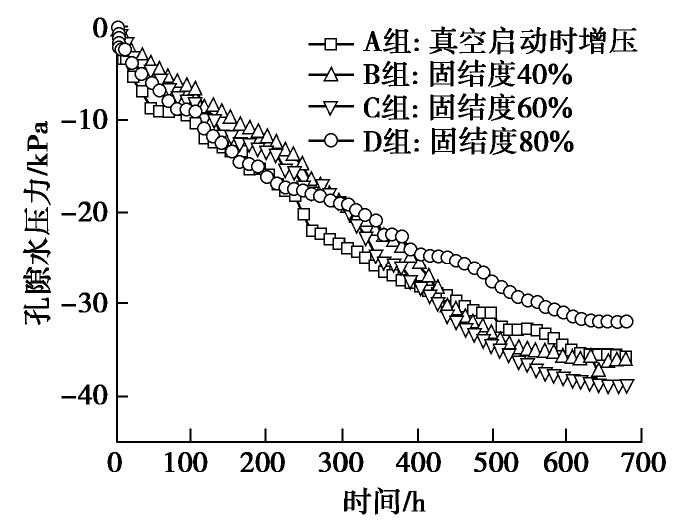
试验编号	加载方式	真空梯度/kPa	加载模式/kPa
1	一级	80	80
2	二级	60	20→80
3	三级	30	20→50→80
4	四级	20	20→40→60→80

下载: 导出CSV

参考文献(97)

[1]	KJELLMAN W. Consolidation of clay soil by means of atmospheric pressure[C]//Proc Conf on Soil Stabilization, MIT. 1952: 258-263.
[2]	HOLTZ R D, JAMIOLKOWSKI M B, LANCELLOTTA R, et al. Prefabricated Vertical Drains: Design and Performance[M]. 1991.
[3]	BERGADO D T, MANIVANNAN R, BALASUBRAMANIAM A S. Filtration criteria for prefabricated vertical drain geotextile filter jackets in soft Bangkok clay[J]. Geosynthetics International, 1996, 3(1): 63-83. doi: 10.1680/gein.3.0054
[4]	CHU J, YAN S W, YANG H. Soil improvement by the vacuum preloading method for an oil storage station[J]. Géotechnique, 2000, 50(6): 625-632. doi: 10.1680/geot.2000.50.6.625
[5]	YAN S W, CHU J. Soil improvement for a storage yard using the combined vacuum and fill preloading method[J]. Canadian Geotechnical Journal, 2005, 42(4): 1094-1104. doi: 10.1139/t05-042
[6]	SHEN S L, CHAI J C, HONG Z S, et al. Analysis of field performance of embankments on soft clay deposit with and without PVD-improvement[J]. Geotextiles and Geomembranes, 2005, 23(6): 46-485.
[7]	CHAI J, MIURA N, BERGADO D T. Preloading clayey deposit by vacuum pressure with cap-drain: analyses versus performance[J]. Geotextiles and Geomembranes, 2008, 26(3): 220-230. doi: 10.1016/j.geotexmem.2007.10.004
[8]	CHAI J C, HONG Z S, SHEN S L. Vacuum-drain consolidation induced pressure distribution and ground deformation[J]. Geotextiles and Geomembranes, 2010, 28(6): 525-535. doi: 10.1016/j.geotexmem.2010.01.003
[9]	FU H, CAI Y, WANG J, et al. Experimental study on the combined application of vacuum preloading-variable-spacing electro-osmosis to soft ground improvement[J]. Geosynthetics International, 2017, 24(1): 72-81. doi: 10.1680/jgein.16.00016
[10]	CAI Y Q, XIE Z W, WANG J, et al. New approach of vacuum preloading with booster prefabricated vertical drains (PVDs) to improve deep marine clay strata[J]. Canadian Geotechnical Journal, 2018, 55(10): 1359-1371. doi: 10.1139/cgj-2017-0412
[11]	ABUEL-NAGA H M, BERGADO D T, GNIEL J. Design chart for prefabricated vertical drains improved ground[J]. Geotextiles and Geomembranes, 2015, 43(6): 537-546. doi: 10.1016/j.geotexmem.2015.04.021
[12]	ZHOU Y, CHAI J C. Equivalent ‘smear’ effect due to non-uniform consolidation surrounding a PVD[J]. Géotechnique, 2017, 67(5): 410-419. doi: 10.1680/jgeot.16.P.087
[13]	WANG P, HAN Y, ZHOU Y, et al. Apparent clogging effect in vacuum-induced consolidation of dredged soil with prefabricated vertical drains[J]. Geotextiles and Geomembranes, 2020, 48(4): 524-531. doi: 10.1016/j.geotexmem.2020.02.010
[14]	鲍树峰, 娄炎, 董志良, 等. 新近吹填淤泥地基真空固结失效原因分析及对策[J]. 岩土工程学报, 2014, 36(7): 1350-1359. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201407023.htm BAO Shu-feng, LOU Yan, DONG Zhi-liang, et al. Causes and counter measures for vacuum consolidation failure of newly-dredged mud foundation[J]. Chinese Journal of Geotechnical Engineering, 2014, 36(7): 1350-1359. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201407023.htm
[15]	CAI Y Q, QIAO H H, WANG J, et al. Experimental tests on effect of deformed prefabricated vertical drains in dredged soil on consolidation via vacuumpreloading[J]. Engineering Geology, 2017, 222: 10-19. doi: 10.1016/j.enggeo.2017.03.020
[16]	ZENG L L, CAI Y Q, CUI Y J, et al. Hydraulic conductivity of reconstituted clays based on intrinsic compression[J]. Géotechnique, 2020, 70(3): 268-275. doi: 10.1680/jgeot.18.P.096
[17]	HONG Z S, ONITSUKA K. A method of correcting yieldstress and compression index of Ariake clays for sample disturbance[J]. Soils and Foundations, 1998, 38(2): 211-222. doi: 10.3208/sandf.38.2_211
[18]	施建勇, 雷国辉, 艾英钵, 等. 关于真空预压沉降计算的研究[J]. 岩土力学, 2006, 27(3): 365-368. doi: 10.3969/j.issn.1000-7598.2006.03.005 SHI Jian-yong, LEI Guo-hui, AI Ying-bo, et al. Research of settlement calculation for vacuum preloading[J]. Rock and Soil Mechanics, 2006, 27(3): 365-368. (in Chinese) doi: 10.3969/j.issn.1000-7598.2006.03.005
[19]	LIU J, LEI H, ZHENG G, et al. Laboratory model study of newly deposited dredger fills using improved multiple-vacuum preloading technique[J]. Journal of Rock Mechanics and Geotechnical Engineering, 2017, 9(5): 924-935. doi: 10.1016/j.jrmge.2017.03.003
[20]	ZHAN X J, LIN W A, ZHAN L T, et al. Field implementation of FeCl3-conditioning and vacuum preloading for sewage sludge disposed in a sludge lagoon: a case study[J]. Geosynthetics International, 2015, 22(4): 327-338. doi: 10.1680/gein.15.00015
[21]	NGUYEN T T, INDRARATNA B, RUJIKIATKAMJORN C. An analytical evaluation of radial consolidation with respect to drain degradation[C]//Sixth International Conference on Geotechnique, Construction Materials and Environment, 2016, Bangkok.
[22]	KIM R, HONG S J, LEE M J, et al. Time dependent well resistance factor of PVD[J]. Marine Georesources and Geotechnology, 2011, 29(2): 131-144. doi: 10.1080/1064119X.2010.525145
[23]	CHAI J C, CARTER J P, HAYASHI S. Ground deformation induced by vacuum consolidation[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2005, 131(12): 1552-1561. doi: 10.1061/(ASCE)1090-0241(2005)131:12(1552)
[24]	唐彤芝, 黄家青, 关云飞, 等. 真空预压加固吹填淤泥土现场试验研究[J]. 水运工程, 2010(4): 115-122. doi: 10.3969/j.issn.1002-4972.2010.04.027 TANG Tong-zhi, HUANG Jia-qing, GUAN Yun-fei, et al. Experimental study on dredged fill sludge improved by vacuum preloading[J]. Port & Waterway Engineering, 2010(4): 115-122. (in Chinese) doi: 10.3969/j.issn.1002-4972.2010.04.027
[25]	DENG Y F, LIU L, CUI Y J, et al. Colloid effect on clogging mechanism of hydraulic reclamation mud improved by vacuum preloading[J]. Canadian Geotechnical Journal, 2019, 56(5): 611-620. doi: 10.1139/cgj-2017-0635
[26]	ZHAO C, KOSEKO J, LIU W. Local deformation behaviour of saturated silica sand during undrained cyclic torsional shear tests using image analysis[J]. Géotechnique, 2020, 70(7): 621-629. doi: 10.1680/jgeot.18.T.017
[27]	BOWMAN A J, HAIGH S K. Subsurface deformation mechanisms beneath a flexible pavement using image correlation[J]. Géotechnique, 2019, 69(7): 627-637. doi: 10.1680/jgeot.18.P.092
[28]	FRANZA A, MARSHALL A M, ZHOU B, et al. Greenfield tunnelling in sands: the effects of soil density and relative depth[J]. Géotechnique, 2019, 69(4): 1-25.
[29]	WHITE D J, TAKE W A, BOLTON M D. Soil deformation measurement using particle image velocimetry (PIV) and photogrammetry[J]. Géotechnique, 2003, 53(7): 619-631. doi: 10.1680/geot.2003.53.7.619
[30]	HARDY R A, JAMES M R, PATES J M, et al. Using real time particle tracking to understand soil particle movements during rainfall events[J]. Catena, 2017, 150: 32-38. doi: 10.1016/j.catena.2016.11.005
[31]	CHENG Z, WANG J. A particle-tracking method for experimental investigation of kinematics of sand particles under triaxial compression[J]. Powder Technology, 2018: S003259101731029X.
[32]	LI J F, CHEN H, YUAN X Q, et al. Analysis of the effectiveness of the step vacuum preloading method: a case study on high clay content dredger fill in Tianjin, China[J]. Journal of Marine Science and Engineering, 2020, 8(1): 38. doi: 10.3390/jmse8010038
[33]	FANG Y G, GUO L F, HUANG J W. Mechanism test on inhomogeneity of dredged fill during vacuum preloading consolidation[J]. Marine Georesources& Geotechnology, 2019, 37(8): 1007-1017.
[34]	TERZAGHI K, PECK R B, MESRI G. Soil Mechanics in Engineering Practice[M]. New York: John Wiley & Sons, 1996.
[35]	OUELLETTE N T, XU H, BODENSCHATZ E. A quantitative study of three-dimensional Lagrangian particle tracking algorithms[J]. Experiments in Fluids, 2006, 40(2): 301-313. doi: 10.1007/s00348-005-0068-7
[36]	LIU S J, CAI Y Q, SUN H L, et al. Consolidation considering clogging effect under uneven strain assumption[J]. International Journal of Geomechanics, 2021, 21(1): 04020239. doi: 10.1061/(ASCE)GM.1943-5622.0001898
[37]	蔡袁强, 周岳富, 王鹏, 等. 考虑淤堵效应的疏浚淤泥真空固结沉降计算[J]. 岩土力学, 2020, 41(11): 1-10. doi: 10.16285/j.rsm.2020.0262 CAI Yuan-qiang, ZHOU Yue-fu, WANG Peng, et al. Calculation on settlement of dredged slurry treated by vacuum preloading method with consideration of clogging effects[J]. Rock and Soil Mechanics, 2020, 41(11): 1-10. (in Chinese) doi: 10.16285/j.rsm.2020.0262
[38]	沈杰. 高含水率疏浚泥真空预压室内模型试验研究[D]. 南京: 东南大学, 2015. SHEN Jie. Laboratory Model Test of Vacuum Preloading on Dredged Clays at High Initial Water Contents[D]. Nanjing: Southeast University, 2015. (in Chinese)
[39]	娄晨晖. 排水板与初始含水率对真空预压加固吹淤泥效果及影响范围试验研究[D]. 温州: 温州大学, 2019. LOU Chen-hui. Experimental on the Effect of PVDs and Initial Water Content on Vacuum Preloading Reinforcement and Range of Drainage Dredger Fill[D]. Wenzhou: Wenzhou University, 2019. (in Chinese)
[40]	WANG J, CAI Y Q, MA J J, et al. Improved vacuum preloading method for consolidation of dredged clay-slurry fill[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2016, 142(11): 1-5.
[41]	程建远, 袁俊俊. 不同排水板布置方式真空预压现场试验研究[J]. 中国水运(下半月), 2020, 20(4): 259-261. https://www.cnki.com.cn/Article/CJFDTOTAL-ZSUX202004110.htm CHEN Jian-yuan, YUAN Jun-jun. Field test on vacuum preloading with different drainage arrangements[J]. China Water Transport, 2020, 20(4): 259-261. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZSUX202004110.htm
[42]	BARRON RA. Consolidation of fine-grained soils by drain wells[J]. ASCE Transactions, 1948, 113: 718-754.
[43]	谢康和, 曾国熙. 等应变条件下的砂井地基固结解析理论[J]. 岩土工程学报, 1989, 11(2): 3-17. doi: 10.3321/j.issn:1000-4548.1989.02.002 XIE Kang-he, ZENG Guo-xi. Consolidation theories for drain wells under equal strain condition[J]. Chinese Journal of Geotechnical Engineering, 1989, 11(2): 3-17. (in Chinese) doi: 10.3321/j.issn:1000-4548.1989.02.002
[44]	沈珠江, 陆舜英. 软土地基真空排水预压的固结变形分析[J]. 岩土工程学报, 1986, 8(3): 7-15. doi: 10.3321/j.issn:1000-4548.1986.03.002 SHEN Zhu-jiang, LU Shun-ying. Analysis of consolidation and deformation of soft subsoil under vacuum[J]. Chinese Journal of Geotechnical Engineering, 1986, 8(3): 7-15. (in Chinese) doi: 10.3321/j.issn:1000-4548.1986.03.002
[45]	HANSBO S. Consolidation of fine‐grained soils by prefabricated drains[C]//Proc 10th International Conference on Soil Mechanics and Foundation Engineering, 1981, Stockholm.
[46]	GIBSON R E, SCHIFFMAN R L, CARGILL K W. The theory of one-dimensional consolidation of saturated clays: II finite nonlinear consolidation of thick homogeneous layers[J]. Canadian Geotechnical Journal, 1981, 18(2): 280-293. doi: 10.1139/t81-030
[47]	YOSHIKUNI H, NAKANODO H. Consolidation of soils by vertical drain wells with finite permeability[J]. Soils and Foundations, 1974, 14(2): 35-46. doi: 10.3208/sandf1972.14.2_35
[48]	LEI H, LU H, LIU J, et al. Experimental study of the clogging of dredger fills under vacuum preloading[J]. International Journal of Geomechanics, 2017, 17(12): 04017117. doi: 10.1061/(ASCE)GM.1943-5622.0001028
[49]	TERZAGHI , KARL . Theoretical Soil Mechanics[M]. New York: John Wiley & Sons, Inc, 1943.
[50]	GIBSON R E, ENGLAND G L, HUSSEY M J L. The Theory of one-dimensional consolidation of saturated clays: I finite non-linear consildation of thin homogeneous layers[J]. Géotechnique, 1967, 17(3): 261-273. doi: 10.1680/geot.1967.17.3.261
[51]	江辉煌, 赵有明, 刘国楠, 等. 砂井地基的大变形固结[J]. 岩土工程学报, 2011, 33(2): 302-308. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201102026.htm JIANG Hui-huang, ZHAO You-ming, LIU Guo-nan, et al. Large strain consolidation of soft ground with vertical drains[J]. Chinese Journal of Geotechnical Engineering, 2011, 33(2): 302-308. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201102026.htm
[52]	GENG X, YU H S. A large-strain radial consolidation theory for soft clays improved by vertical drains[J]. Géotechnique, 2017, 67(11): 1020-1028. doi: 10.1680/jgeot.15.T.013
[53]	鲍树峰, 莫海鸿, 董志良, 等. 新近吹填淤泥地基负压传递特性及分布模式研究[J]. 岩土力学, 2014, 35(12): 3569-3576. doi: 10.16285/j.rsm.2014.12.034 BAO Shu-feng, MO Hai-hong, DONG Zhi-liang, et al. Research on transfer properties and distribution model of negativepressure in fresh hydraulic reclamation muck foundation[J]. Rock and Soil Mechanics, 2014, 35(12): 3569-3576. (in Chinese) doi: 10.16285/j.rsm.2014.12.034
[54]	DENG Y, LIU L, CUI Y J, et al. Colloid effect on clogging mechanism of hydraulic reclamation mud improved by vacuum preloading[J]. Canadian Geotechnical Journal, 2019, 56(5): 611-620. doi: 10.1139/cgj-2017-0635
[55]	CHAI J C, MIURA N. Investigation of factors affecting vertical drain behavior[J]. Journal of Geotechnical and Geoenvironmental Engineering, 1999, 125(3): 216-226. doi: 10.1061/(ASCE)1090-0241(1999)125:3(216)
[56]	沈洪忠. 低位真空预压软土地基加固技术在温州的应用[J]. 市政技术, 2003(3): 35-38. https://www.cnki.com.cn/Article/CJFDTOTAL-SZJI200303004.htm SHEN Hong-zhong. Application of low-position vacuum preloading soft soil foundation reinforcement technology in Wenzhou[J]. Municipal Technology, 2003(3): 35-38. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SZJI200303004.htm
[57]	李丽慧. 立体式真空降水法分层加固吹填土的可行性研究[J]. 岩土工程学报, 2002, 24(4): 522-524. doi: 10.3321/j.issn:1000-4548.2002.04.025 LI Li-hui. The feasibility study of three-dimensional vacuum precipitation method to strengthen dredger fill in layers[J]. Chinese Journal of Geotechnical Engineering. 2002, 24(4): 522-524. (in Chinese) doi: 10.3321/j.issn:1000-4548.2002.04.025
[58]	韦剑锋. 天津市滨海新区吹填土工程处理现状及技术改进试验研究——吹填土水平辐射真空排水固结技术初探[J]. 工程勘察, 2008(6): 20-23. https://www.cnki.com.cn/Article/CJFDTOTAL-GCKC200806009.htm WEI Jian-feng. Tianjin Binhai New area dredger fill engineering treatment status quo and technical improvement test research: a preliminary study on horizontal radiation vacuum drainage consolidation technology of dredger fill[J]. Engineering Investigation, 2008(6): 20-23. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GCKC200806009.htm
[59]	刘松玉, 韩文君, 章定文, 等. 劈裂真空法加固软土地基试验研究[J]. 岩土工程学报, 2012, 34(4): 591-599. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201204005.htm LIU Song-yu, HAN Wen-jun, ZHANG Ding-yi, et al. Experimental study on soft soil foundation reinforcement by split vacuum method[J]. Chinese Journal of Geotechnical Engineering, 2012, 34(4): 591-599. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201204005.htm
[60]	YUAN X Q, WANG Q, CHEN H. Laboratory Test on Dredger Fill Reinforced by Hierarchical Vacuum Preloading[C]//Multimedia Technology (ICMT), 2011 International Conference, 2011, Hangzhou.
[61]	苑晓青, 王清, 孙铁. 分级真空预压法加固吹填土过程中孔隙分布特征[J]. 吉林大学学报(地球科学版), 2012, 42(1): 169-176. doi: 10.13278/j.cnki.jjuese.2012.01.013 YUAN Xiao-qing, WANG Qing, SUN Tie. Pore distribution characteristics in the process of consolidation of dredger fill with stepped vacuum preloading method[J]. Journal of Jilin University (Earth Science Edition), 2012, 42(1): 169-176. (in Chinese) doi: 10.13278/j.cnki.jjuese.2012.01.013
[62]	LEI H Y, QI Z Y, ZHANG Z P. New vacuum-preloading technique for ultrasoft-soil foundations using model tests[J]. International Journal of Geomechanics, ASCE, 2017, 17(9): 04017049. doi: 10.1061/(ASCE)GM.1943-5622.0000934
[63]	LIU J J, LEI H Y, FENG S X, et al. Improved synchronous and alternate vacuum preloading method on newly dredger fills: laboratory model study[J]. International Journal of Geomechanics, ASCE, 2018, 18(8): 04018086. doi: 10.1061/(ASCE)GM.1943-5622.0001220
[64]	孙立强, 闫澍旺, 李伟, 等. 超软土真空预压室内模型试验研究[J]. 岩土力学, 2011, 32(4): 984-990. doi: 10.3969/j.issn.1000-7598.2011.04.005 SUN Li-qiang, YAN Shu-wang, LI Wei, et al. Study of super-soft soil vacuum preloading model test[J]. Rock and Soil Mechanics, 2011, 32(4): 984-990. (in Chinese) doi: 10.3969/j.issn.1000-7598.2011.04.005
[65]	WANG J, CAI Y Q, FU H T, et al. Experimental study on the dredged fill ground improved by a two-staged vacuum preloading method[J]. Soils and Foundations, 2018, 58(3): 766-775. doi: 10.1016/j.sandf.2018.02.028
[66]	ZHEN G, YAN X, ZHOU H, et al. Effects of calcined aluminum salts on the advanced dewatering and solidification/stabilization of sewage sludge[J]. Journal of Environmental Science, 2011, 23(7): 1225-1232. doi: 10.1016/S1001-0742(10)60539-6
[67]	THAPA K B, QI Y, CLAYTON S A, et al. Lignite aided dewatering of digested sewage sludge[J]. Water Resources 2009, 43: 623-634.
[68]	刘莹, 王清. 水泥与生石灰处理吹填土对比试验研究[J]. 工程地质学报, 2006, 14(3): 424-429. doi: 10.3969/j.issn.1004-9665.2006.03.026 LIU Ying, WANG Qing. Laboratory model tests on consolidation of hydraulic fills treated with lime or cement additives[J]. Journal of Engineering Geology, 2006, 14(3): 424-429. (in Chinese) doi: 10.3969/j.issn.1004-9665.2006.03.026
[69]	胡挺, 武亚军, 徐方. 氢氧化钙与聚丙烯酰胺对高含水率淤泥固结的影响[J]. 长江科学院院报, 2018, 35(8): 95-101. https://www.cnki.com.cn/Article/CJFDTOTAL-CJKB201808025.htm HU Ting, WU Ya-jun, XU Fang. Effect of calcium hydroxide and anionic polyacrylamide on consolidation of slurry of high water content[J]. Journal of Yangtze Riverscientific Research Institute, 2018, 35(8): 95-101. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-CJKB201808025.htm
[70]	QUANG N D, CHAI J C. Permeability of lime-and cement-treated clayey soils[J]. Canadian Geotechnical Journal, 2015, 52(9): 1221-1227. doi: 10.1139/cgj-2014-0134
[71]	武亚军, 陆逸天, 牛坤, 等. 药剂真空预压法处理工程废浆试验[J]. 岩土工程学报, 2016, 38(8): 1365-1373. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201608002.htm WU Ya-jun, LU Yi-tian, NIU Kun, et al. Experimental study on solid-liquid separation of construction waste slurry by additive agent-combined vacuum preloading[J]. Chinese Journal of Geotechnical Engineering, 2016, 38(8): 1365-1373. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201608002.htm
[72]	WU Y J, KONG G Q, LU Y T, et al. Experimental study on vacuum preloading with flocculation in waste slurry improvement[J]. Geomechanics and Engineering. 2017, 13(2): 319-331.
[73]	LEI H Y, XU Y G, LI X, et al. Effect of polyacrylamide on improvement of dredger fill with vacuum preloading method[J]. Journal of Materials in Civil Engineering, 2019, 31(9): 04019193. doi: 10.1061/(ASCE)MT.1943-5533.0002860
[74]	CAI Y Q, QIAO H H, WANG J, et al. Experimental tests on effect of deformed prefabricate dverticaldrainsindredged soil on con soli dationvia vacuum preloading[J]. Engineering Geology, 2017, 222: 10-19. doi: 10.1016/j.enggeo.2017.03.020
[75]	王宏伟. 不同排水板滤膜在地基固结中的对比研究[D]. 天津: 天津大学, 2009. WANG Hong-wei. Comparative Study of Different Drainage Plate Filter Membranes in Foundation Consolidation[D]. Tianjin: Tianjin University, 2009. (in Chinese)
[76]	丁长生. 天津临港工业区真空预压试验及塑料排水板保土性研究[D]. 天津: 天津大学, 2012. DING Chang-sheng. Vacuum Preloading Test of Tianjin Lingang Industrial Zone and Research On Soil Retention of Plastic Drainage Board[D]. Tianjin: Tianjin University, 2012. (in Chinese)
[77]	WANG J, YANG Y L, FU H T, et al. Improving consolidation of dredged slurry by vacuum preloading using PVDs with varying filter pore sizes[J]. Canadian Geotechnical Journal, 2020, 57(2): 294-303. doi: 10.1139/cgj-2018-0572
[78]	乐超, 徐超, 吴雪峰, 等. 两种塑料排水板滤膜淤堵特性试验研究[J]. 岩土力学, 2014, 35(9): 2529-2534. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201409014.htm LE Chao, XU Chao, WU Xue-feng, et al. Experimental research on clogging characteristic of two types of PVD filters[J]. Rock and Soil Mechanics, 2014, 35(9): 2529-2534. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201409014.htm
[79]	CARROLL R G. Geotextile Filter Criteria[R]. Washington D C: Transportation Research Board, 1983: 46-53.
[80]	CHU J, BO M W, CHOA V. Practical considerations for using vertical drains in soil improvement projects[J]. Geotextiles & Geomembranes, 2004, 22(1): 101-117.
[81]	American Society for Testing and Materials(ASTM). Standard Test Method for Measuring the Soilgeotextile Clogging Potential by the Gradient Ratio: D5101-01[S]. 2003.
[82]	INDRARATNA B, SATHANANTHAN I, RUJIKIATKAMJORN C, et al. Analytical and numerical modeling of soft soil stabilized by prefabricated vertical drains incorporating vacuum preloading[J]. International Journal of Geomechanics, 2005, 5(2): 114-124. doi: 10.1061/(ASCE)1532-3641(2005)5:2(114)
[83]	徐锴, 林生法, 耿之周. 真空加载方式对排水板滤膜淤堵影响试验研究[J]. 岩土工程学报, 2016, 38(增刊2): 123-129. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC2016S2020.htm XU Kai, LIN Sheng-fa, GENG Zhi-zhou. Experimental study on the influence of vacuum loading method on the clogging of drainage plate filter membrane[J]. Chinese Journal of Geotechnical Engineering, 2016, 38(S2): 123-129. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC2016S2020.htm
[84]	LIU J J, LEI H Y, ZHENG G. Laboratory model study of newly deposited dredger fills using improved multiple-vacuum preloading technique[J]. Journal of Rock Mechanics and Geotechnical Engineering, 2017, 9(5): 924-935. doi: 10.1016/j.jrmge.2017.03.003
[85]	金亚伟, 金亚军, 蒋君南, 等. 增压式真空预压固结处理软土地基/尾矿渣/湖泊淤泥的方法: CN101418566[P]. 2009-04-29. JIN Ya-wei, JIN Ya-jun, JIANG Jun-nan, et al. The Treatment Method for Soft Soil Foundation/Tailing Slag/Lake Silt by Air-Booster Vacuum Preloading: CN101418566[P]. 2009-04-29. (in Chinese)
[86]	朱平, 孙立强, 闫澍旺, 等. 可控通气真空预压室内模型试验及其机制分析[J]. 岩石力学与工程学报, 2011, 30(增刊1): 3041-3148. https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX2011S1072.htm ZHU Ping, SUN Li-qiang, YAN Shu-wang, et al. Laboratory model test and mechanism analysis of controlled ventilation vacuum precompression[J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(S1): 3041-3148. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX2011S1072.htm
[87]	沈宇鹏, 余江, 刘辉, 等. 增压式真空预压处理站场软基效果试验研究[J]. 铁道学报, 2011, 33(5): 97-103. https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB201105023.htm SHEN Yu-peng, YU Jiang, LIU Hui, et al. Experimental study on the effect of pressurized vacuum preloading treatment of station yard soft foundation[J]. Journal of the China Railway Society, 2011, 33(5): 97-103. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TDXB201105023.htm
[88]	沈宇鹏, 冯瑞玲, 余江, 等. 增压式真空预压处理软基的加固机理[J]. 吉林大学学报(地球科学版), 2012, 42(3): 792-797. https://www.cnki.com.cn/Article/CJFDTOTAL-CCDZ201203024.htm SHEN Yu-peng, FENG Rui-ling, YU Jiang, et al. Strengthening mechanism of pressurized vacuum preloading treatment of soft foundation[J]. Journal of Jilin University (Earth Science Edition), 2012, 42(3): 792-797. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-CCDZ201203024.htm
[89]	LIN W A, ZHAN X, ZHAN T L, et al. Effect of FeCl₃-conditioning on consolidation property of sewage sludge and vacuum preloading test with integrated PVDs at the Changan landfill, China[J]. Geotextiles and Geomembranes, 2014, 42: 181-190.
[90]	LIU F Y, WU W Q, FU H T, et al. Application of flocculation combined with vacuum preloading to reduce river-dredged sludge[J/OL]. Marine Georesources& Geotechnology, 2019. doi: 10.1080/1064119X.2018.1564092.
[91]	WANG J, NI J F, CAI Y Q, et al. Combination of vacuum preloading and lime treatment for improvement of dredged fill[J]. Engineering Geology, 2017, 227: 149-158.
[92]	RAJASEKARAN G, RAO S N. Permeability characteristics of lime treated marine clay[J]. Ocean Engineering, 2002, 29(2): 113-127.
[93]	ALHASSAN M. Permeability of lateritic soil treated with lime and rice husk ash[J]. Assumption University Journal of Thailand, 2008, 12(2): 115-120.
[94]	ONITSUKA K, MODMOLTIN C, KOUNO M. Investigation on microstructure and strength of lime and cement stabilized Ariake clay[J]. Rep Fac Sci Eng Saga Univ, 2001, 30: 49-63.
[95]	LE RUNIGO B, CUISINER O, CUI Y J, et al. Impact of initial state on the fabric and permeability of alime-treated silt under long-term leaching[J]. Canadian Geotechnical Journal, 2009, 46(11): 1243-1257.
[96]	RAJASEKARAN G, MURALI K, SRINIVASARAGHAVAN R. Fabric and mineralogicalstudies on lime treated marine clays[J]. Ocean Engineering, 1997, 24(3): 227-234.
[97]	WANG J, HUANG G, FU H T, et al. Vacuum preloading combined with multiple-flocculant treatment for dredged fillimprovement[J]. Engineering Geology, 2019, 259: 105194.